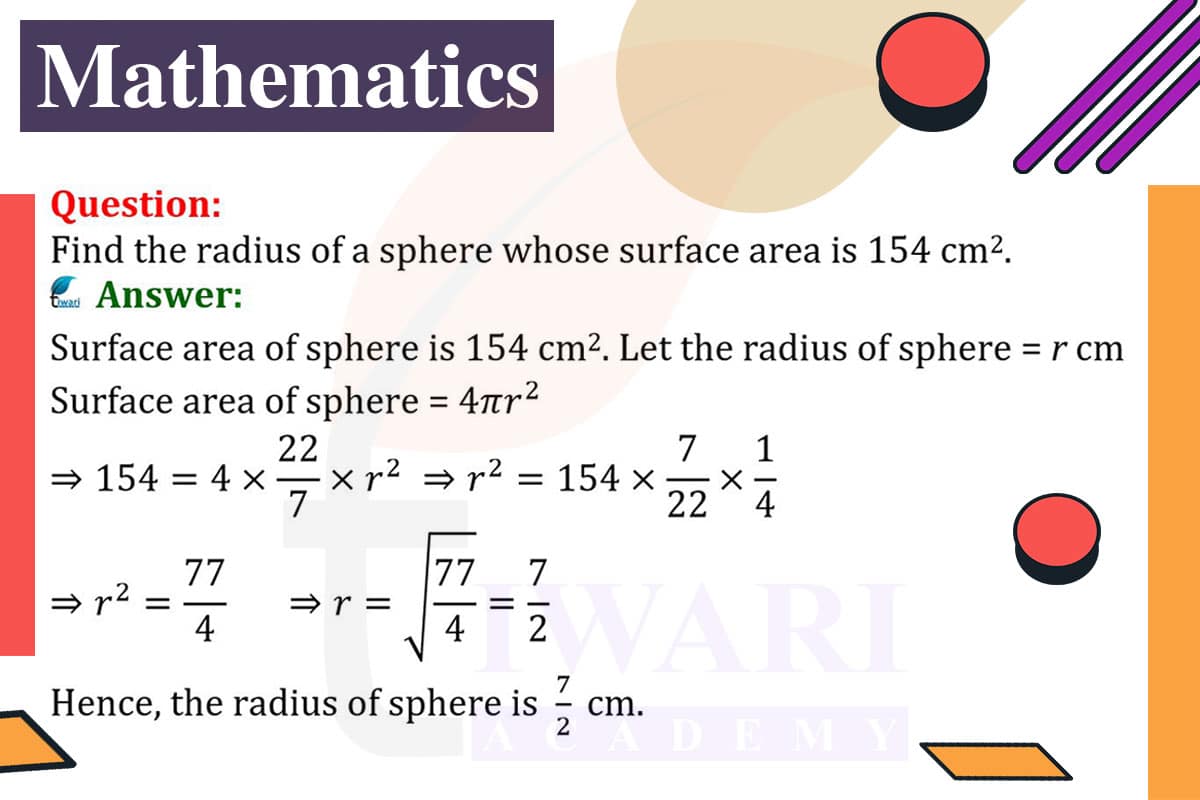
To find the radius of a sphere with a given surface area, we use the formula for the surface area of a sphere, which is 4πr², where r is the radius. Given that the surface area is 154 cm², we set up the equation 4πr² = 154.
Rearranging the formula to solve for r² = 154/4π. Using the approximation π ≈ 3.14, the equation becomes r² = 154/(4 × 3.14). Solving this, we find r² ≈ 12.26, and thus r ≈ √12.26, which is approximately 3.5 cm. Therefore, the radius of the sphere is about 3.5 cm. This calculation is a straightforward application of algebra to geometric formulas, illustrating how mathematical principles are used to derive dimensions from known properties of shapes.
Let’s discuss in detail
Determining Sphere Radius from Surface Area
The task of finding the radius of a sphere when given its surface area is a classic problem in geometry, illustrating the practical application of algebraic manipulation in solving geometric problems. The surface area of a sphere is given by the formula 4πr², where r is the radius. In this scenario, we are provided with a surface area of 154 cm² and are required to work backwards to determine the sphere’s radius, showcasing the interplay between algebra and geometry.
Understanding the Surface Area Formula
The surface area of a sphere is calculated using the formula 4πr². This formula encapsulates how the surface area is directly proportional to the square of the radius. The constant 4π is derived from the geometric properties of a sphere, representing the relationship between a sphere’s radius and its surface area. This formula is fundamental in geometry and is widely used in various fields, from physics to engineering, where understanding the properties of spherical objects is essential.
Setting up the Equation
To find the radius, we start by setting up the equation with the given surface area: 4πr² = 154 cm². This equation forms the basis for our calculation. The goal is to isolate r (the radius) on one side of the equation, allowing us to solve for it. This process involves algebraic manipulation, showcasing how algebra is used to solve geometric problems.
Algebraic Manipulation to Solve for Radius
The next step involves rearranging the equation to solve for r. We divide both sides of the equation by 4π to isolate r², resulting in r² = 154/4π. Using the approximation π ≈ 3.14, the equation simplifies to r² ≈ 154/(4 × 3.14). This step reduces the problem to a basic algebraic equation, making it easier to solve for r.
Calculating the Radius
Solving the simplified equation, we find r² ≈ 12.26. To find the radius r, we take the square root of this value, resulting in r ≈ √12.26. This calculation yields a radius of approximately 3.5 cm. This final step of taking the square root is crucial as it converts the squared radius back to the actual radius, providing the solution to our problem.
This exercise demonstrates the practical application of algebra in solving a geometric problem, specifically in determining the dimensions of a shape based on its properties. The ability to calculate the radius of a sphere from its surface area has significant implications in various fields, including engineering, design, and physics. It highlights the importance of mathematical principles in understanding and solving real-world problems, emphasizing the interconnectedness of different areas of mathematics.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.2 in Detail


