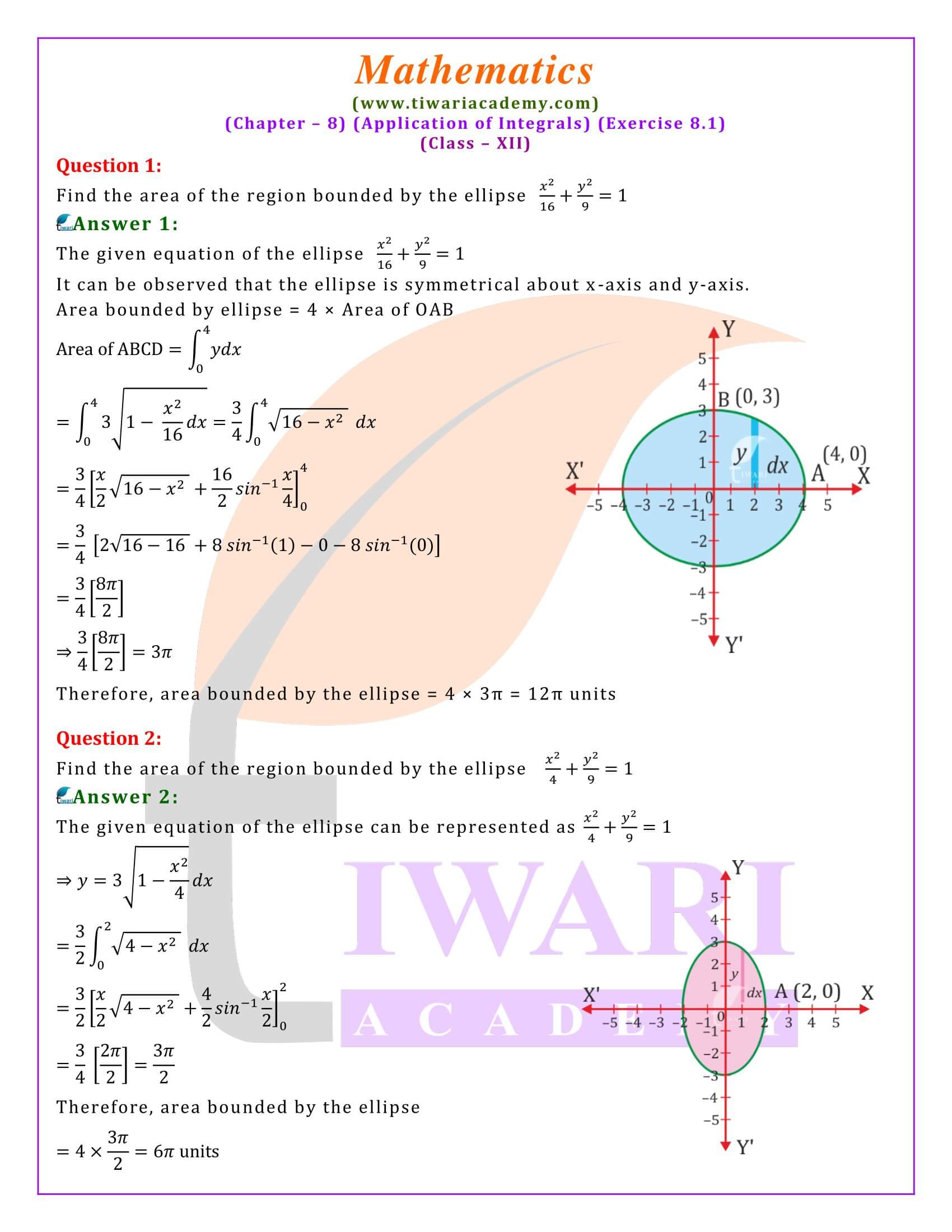
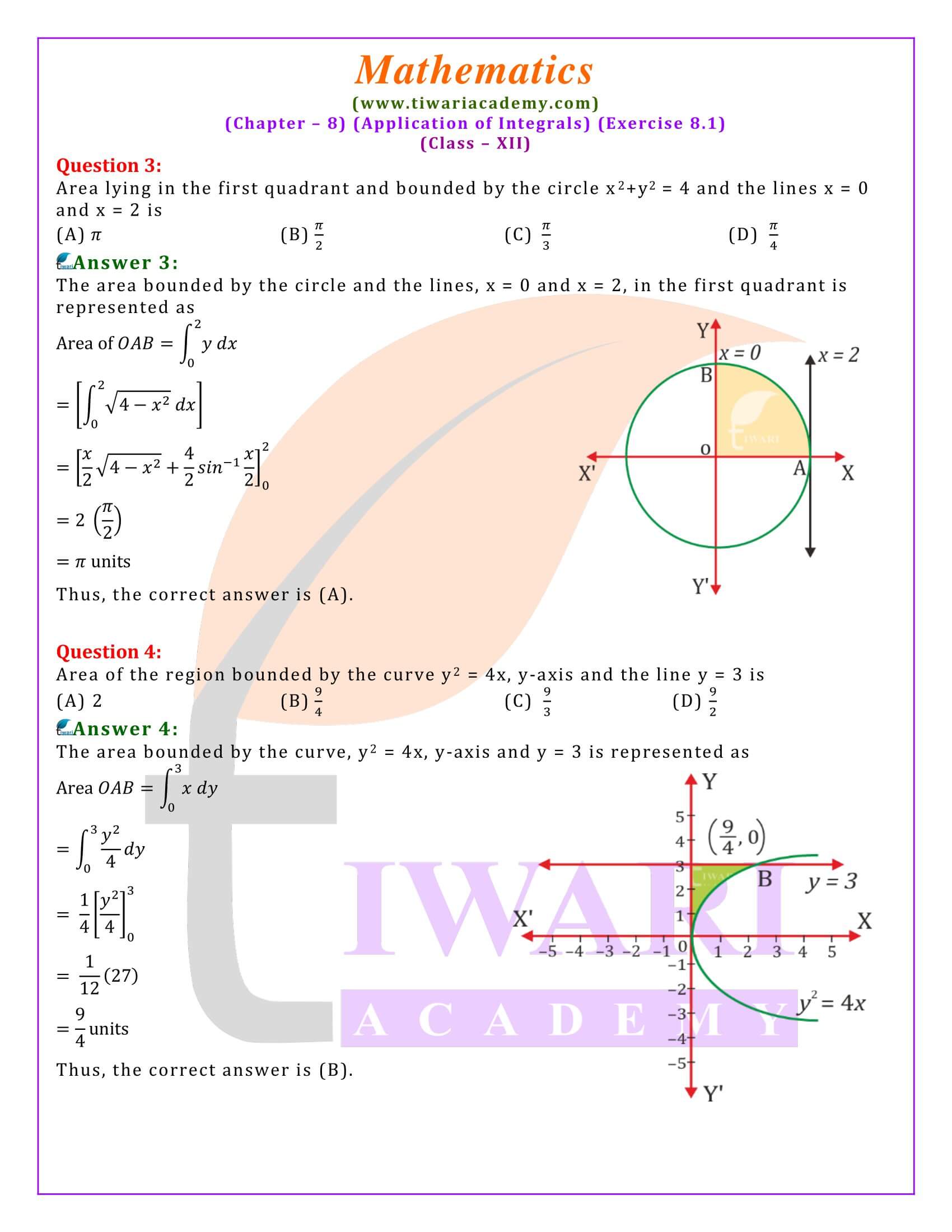
NCERT Solutions for Class 12 Maths Exercise 8.1 Application of Integrals in Hindi and English Medium for CBSE exams 2024-25. The question and answers of ex. 8.1 class 12 Maths are modified as per the new rationalised syllabus and latest NCERT textbooks for CBSE 2024-25.
Class 12 Maths Exercise 8.1 in Hindi and English Medium
Class 12 Maths Chapter 8 Exercise 8.1 Solution
All the contents and video solutions are available in Hindi Medium as well as English Medium updated for academic session 2024-25. CBSE, UP board, MP board and other state boards, who are using NCERT Books for current academic year. can take the benefits of these solution for understanding the concepts of questions given in exercise 8.1 of class 12 Maths.
| Class: 12 | Mathematics |
| Chapter: 8 | Exercise: 8.1 |
| Chapter Name: | Application of Integrals |
| Content: | Textbook Exercise Solutions |
| Content Mode: | Text and Videos Format |
| Session: | CBSE 2024-25 |
| Medium: | Hindi and English Medium |
Class 12 Maths Exercise 8.1 Solution in Videos
What are applications of Integrals?
We have learned formulas to calculate areas of various geometric shapes in geometry, including triangles, rectangles, trapezoids, and circles. These formulas are fundamental to many real-life problems in applications of mathematics. Formulas for elementary geometry allow us to compute areas of many simple figures. However, they are insufficient for calculating the areas surrounded by curves. For that, we will need some concepts of integral calculus.
In Chapter 7 above, we studied the area bounded by the curve y = f (x), ordinate x = a, x = b, and the x-axis, calculating the definite. In this chapter, we will study a specific application of integrals to find the area under simple curves, the area between lines and arcs, under parabola, and ellipse (standard size only). We will also take care to find the area delimited by these curves.
How do we find Area under the curve?
In Class 12 of Mathematics Chapter 7, we studied the definite integral as the limit of a sum and how to evaluate the definite integral using the fundamental principle of calculus. Now, let us consider an easy and intuitive way to find the area bounded by the curve y = f (x), the x-axis, and the ordinate x = a and x = b. We can think of the area under the curve as having a large number of very thin vertical stripes.
Consider an arbitrary strip of height y and width dx, then dA (area of the initial strip) = ydx, where, y = f (x). This region is called the primary region and is located in an unconstrained position within this region that is specified by some value of x between x and a. We can think of the total area A of the area between the x-axis, as a result of connecting the primary areas of thin strips with the area along x = a, x = b, and the curve y = f (x).
How many questions are there in class 12 Maths exercise 8.1?
Earlier there were total 13 questions in exercise 8.1 of class 12 Maths. But after the implementation of rationalised syllabus, there are only 4 questions in ex. 8.1, in which last two questions are MCQ.
Which question is frequently asked in CBSE exams from exercise 8.1?
Now question number 1 and 2 are important as per examination point of view. As compared to other questions, question number 1 and 2 are not tricky but important for CBSE exams.
Which examples is known as most important example in exercise 8.1 class 12 Math?
There are only a few examples and all are basic questions to learn how to find the area bounded between the curves.