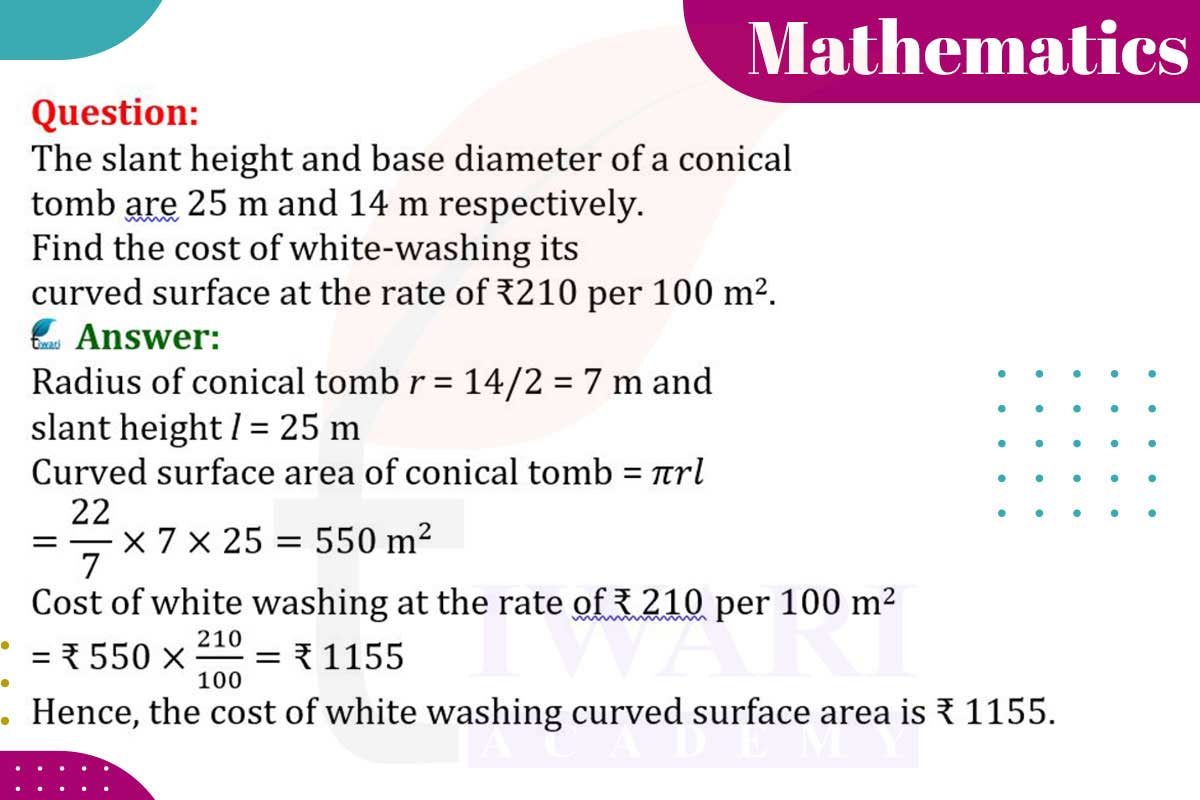
To find the cost of white-washing the curved surface of a conical tomb with a slant height of 25 m and a base diameter of 14 m, first calculate the radius (7 m). The curved surface area is 3.14 × 7 × 25 = 549.5 m². The cost per 100 m² is ₹210, so for 549.5 m², the cost is (549.5/100) × 210 ≈ ₹1153.95.
Introduction to Conical Tomb White-Washing
White-washing a conical tomb involves calculating the surface area to be painted and estimating the cost based on a given rate. In this scenario, we are tasked with determining the cost of white-washing a conical tomb with specific dimensions. The tomb has a slant height of 25 meters and a base diameter of 14 meters. The cost of white-washing is given as ₹210 per 100 square meters. This task requires an application of geometric formulas and basic arithmetic to arrive at the total cost.
Understanding the Geometry of the Tomb
The tomb in question is conical, a shape characterized by a circular base and a pointed top. The slant height is the distance from the top of the tomb to the edge of the base, measured along the surface. The diameter of the base is the total distance across the circular base. For our calculation, we need to first determine the radius of the base, which is half the diameter.
Calculating the Radius of the Base
The radius of the base is a crucial measurement for calculating the surface area. Since the diameter of the tomb’s base is 14 meters, the radius is half of this, which is 7 meters. The radius is used in the formula for calculating the curved surface area of the cone, which is the area that needs to be white-washed.
Determining the Curved Surface Area
The curved surface area of the tomb is calculated using the formula for the surface area of a cone, which is πrl, where r is the radius and l is the slant height. Substituting the values we have, 3.14 × 7 × 25, we get a curved surface area of approximately 549.5 square meters. This is the total area that requires white-washing.
Calculating the White-Washing Cost
The cost of white-washing is given as ₹210 per 100 square meters. To find the total cost for the tomb’s surface area, we divide the total area by 100 and then multiply by the cost per 100 square meters. Therefore, the cost for white-washing 549.5 square meters is (549.5/100) × 210, which amounts to approximately ₹1153.95.
Conclusion and Practical Implications
This calculation demonstrates the practical application of geometry in real-world scenarios, such as construction and maintenance. Accurate estimation of surface area and cost is crucial in budgeting and resource allocation for such projects. The ability to apply mathematical principles to determine the cost of maintaining historical structures like tombs is an essential skill in the fields of architecture, construction, and heritage conservation.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.1 in Detail


