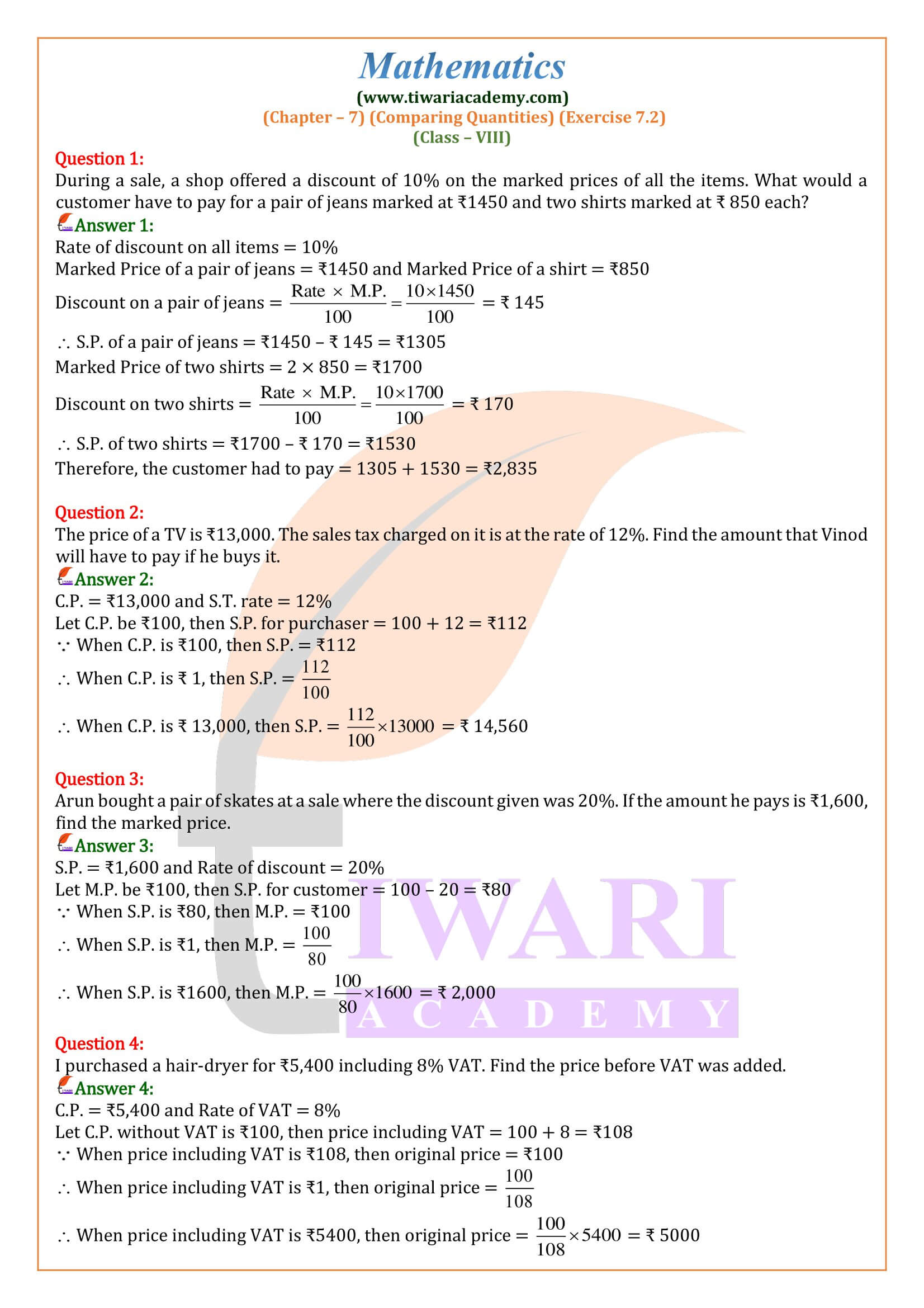
CBSE NCERT Solutions for Class 8 Maths Chapter 7 Exercise 7.2 Comparing Quantities in Hindi and English medium updated for new session 2024-25. Get the revised solution of ex. 7.2 class 8th mathematics based on revised syllabus and new textbooks for CBSE 2024-25 exams.
8th Maths Exercise 7.2 Solution in Hindi and English Medium
| Class: 8 | Mathematics |
| Chapter: 7 | Exercise: 7.2 |
| Topic Name: | Comparing Quantities |
| Content: | NCERT Exercise Solutions |
| Content Type: | Text and Online Videos |
| Session: | CBSE 2024-25 |
| Medium: | Hindi and English Medium |
Class 8 Maths Chapter 7 Exercise 7.2 Solution
Class VIII Mathematics NCERT Chapter 7 Ex. 7.2 Comparing Quantities in PDF file format free to download for academic session 2024-25. Solutions of exercises are available in digital format or PDF and videos format – one for exercises solution and one is for exercises explanation. In class 8 mathematics exercise 7.2, we will discuss about application of percentage, profit and loss based questions and more similar topics.

Profit, Loss and Discount
To calculate Profit, Loss and Discount on a particular material we should have some data like cost price and selling price of material.
Cost price (CP)
The amount for which an article is bought is called its cost price.
Selling price (SP)
The amount for which an article is sold is called its selling price.
Profit or gain
When (SP) > (CP) then there is a gain. Gain = (SP) – (CP).
Loss
When (SP) < (CP) then there is a loss. Loss = (CP) – (SP).
An important fact
The gain or loss is always reckoned on the cost price.
Formulae
- Gain = (SP) – (CP)
- Loss = (CP) – (SP)
- Gain% = {(Gain/CP) x 100} %
- Loss% = {(Loss/CP) x 100} %
To find SP when CP and gain% or loss% are given: (a) SP = {(100 + gain%)/100 x SP} %
(a) SP = {(100 + gain%)/100 x SP} %
(b) SP = {(100 – loss%)/100 x CP} %
To find CP when SP and gain% or loss% are given:
(a) CP = {100/ (100 + gain%) x SP
(b) CP = {100/ (100 – loss%) x SP
Mohit bought a CD for Rs. 750 and sold it for Rs. 875. Find his gain per cent.
CP = Rs. 750 and SP = Rs. 875.
Since (SP) > (CP),
Mohit makes a gain.
Gain = Rs. (875 – 750) = Rs. 125.
Gain% = (gain/CP) x 100 %
= (125/750)/100 %
= 50/3 %
Overheads
Sometimes, after purchasing an article, we have to pay some more money for things like transportation, labour charges, repairing charges, local taxes, etc. These extra expenses are called overheads.
Anuj sells two watches for 1955 each, gaining 15% on one and losing 15% on the other. Find her gain or loss per cent in the whole transaction.
SP of the first watch = 1955
So, CP of the first watch = {100/ (100 + gain%)} x SP
= {100/ (100 + 15)} x 1955 = (100/115) x 1955
= Rs. 1700
SP of the second watch = Rs. 1955
Loss% = 15%.
CP of second watch = {100/ (100 – loss%)} x SP
= (100/85) x 1955
= Rs. 2300
Total CP of the two watches = (1700 + 2300) = 4000.
Total SP of the two watches = (1955 x 2) = 3910.
Since (SP) < (CP), there is a loss in the whole transaction.
Loss = (4000 – 3910) = 90.
So, loss% = (90/4000) x 100 % = 9/4 %
Hence, Anuj loses 9/4 % in the whole transaction
Discount
In order to increase the sale or clear the old stock, sometimes the shopkeepers offer a certain percentage of rebate on the marked price. This rebate is known as discount.
Marked Price
In big shops and departmental stores, every article is tagged with a card and its price is written on it. This is called the marked price of that article, abbreviated as MP.
For books, the printed price is the marked price.
List Price
Items which are manufactured in a factory are marked with a price according to the list supplied by the factory, at which the retailer is supposed to sell them. This price is known as the list price of the article.
An Important Fact: The discount is always reckoned on the marked price.
Selling price = (marked price) – (discount).
The marked price of a ceiling fan is 1250 and the shopkeeper allows a discount of 6% on it. Find the selling price of the fan.
Marked price = 1250 and discount = 6%.
Discount = 6% of MP
= (6% of 1250) = (1250 x 6)/100 = Rs. 75
Selling price = (MP) – (discount) = (1250 – 75) = Rs. 1175.
Sales Tax (ST)
ST is charged by the shopkeeper from the customer on selling price of an item and is added to the value of the bill. Sales tax is paid in a state to the respective state government.
Value Added Tax (VAT)
The Government has to provide various facilities to its citizens and for that it needs money. In order to meet the expenditure, the government imposes certain taxes so as to get the required funds. Value added tax (VAT) is one such tax and it is calculated on the selling price of the goods. VAT or sales tax is levied at a specified rate which is different for different articles and also it differs from state to state.
How do you find the bill amount in exercise 7.2 of 8th Maths if total cost is given?
To find the bill amount using the facts of class 8 Maths exercise 7.2, is easy. To explore this, let us suppose an example:
The cost of a TV set at a showroom was 36500. The sales tax charged was 8% Find the bill amount.
Solution:
Cost of TV set = 36500.
Sales tax = 8% of 36500
= Rs. (36500 x 8/100)
= Rs. 2920
So, bill amount
= (36500 + 2920)
= 39420.
What is use of VAT formula in Exercise 7.2 of Class VIII Mathematics?
To understand the VAT, see the following example:
Raman bought an air cooler for 5400 including VAT at 8%. Find the original price of the air cooler.
Explanation:
Let the original price of the air cooler be x.
VAT = 8% of x = Rs. (x X 8/100) = Rs. 2x/25
So, Price including VAT = Rs. (x + 2x/25) = Rs. 27x/25
So, 27x/25 = 5400
X = 5400 x 25/27 = 5000
Hence, the original price of the air cooler is 5000.
What is the formula of marked price in class 8 Maths exercise 7.2?
This is basically labelled by shopkeepers to offer a discount to the customers in such a way that,
Discount = Marked Price – Selling Price.
Discount Percentage = (Discount/Marked price) x 100.