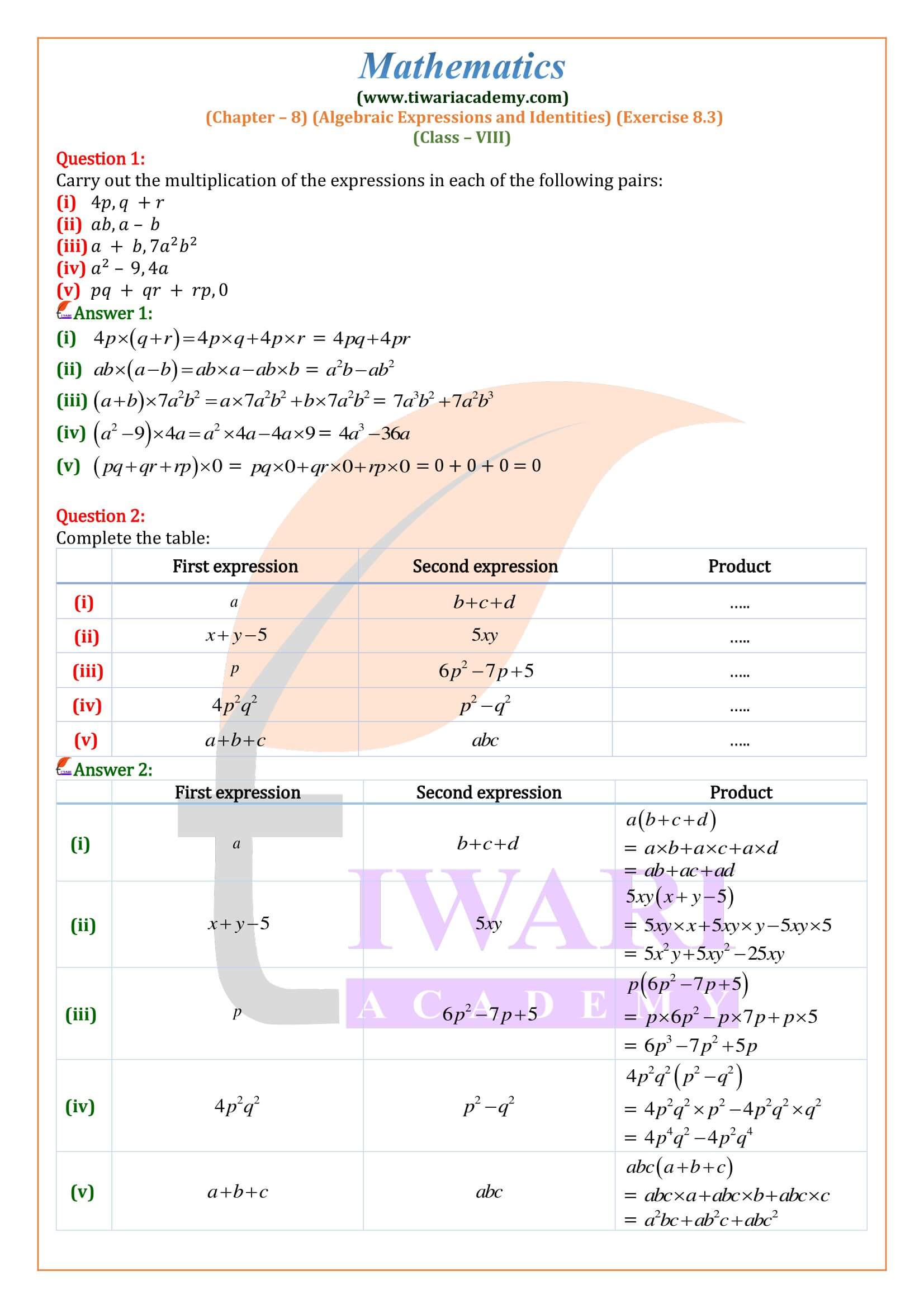
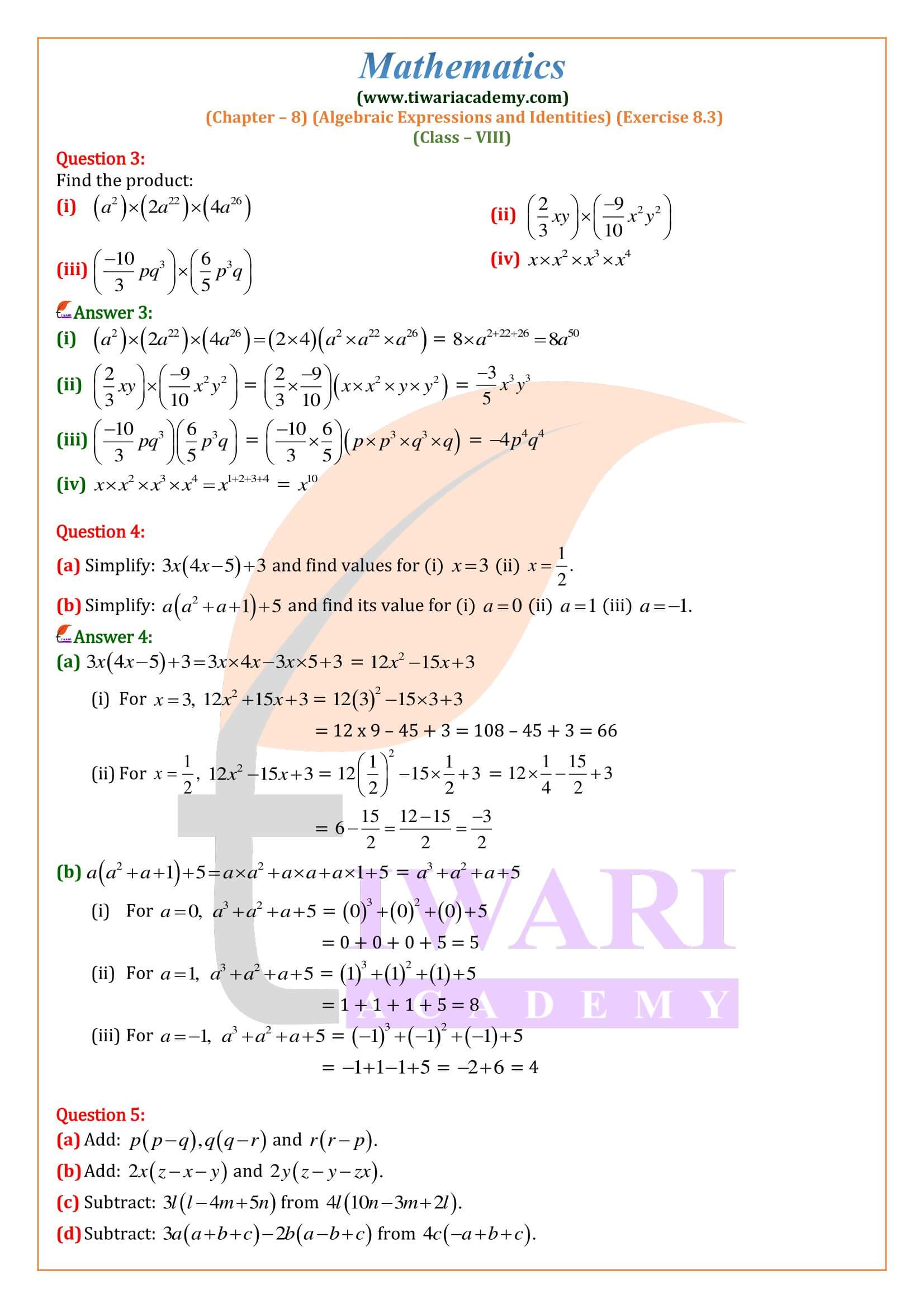
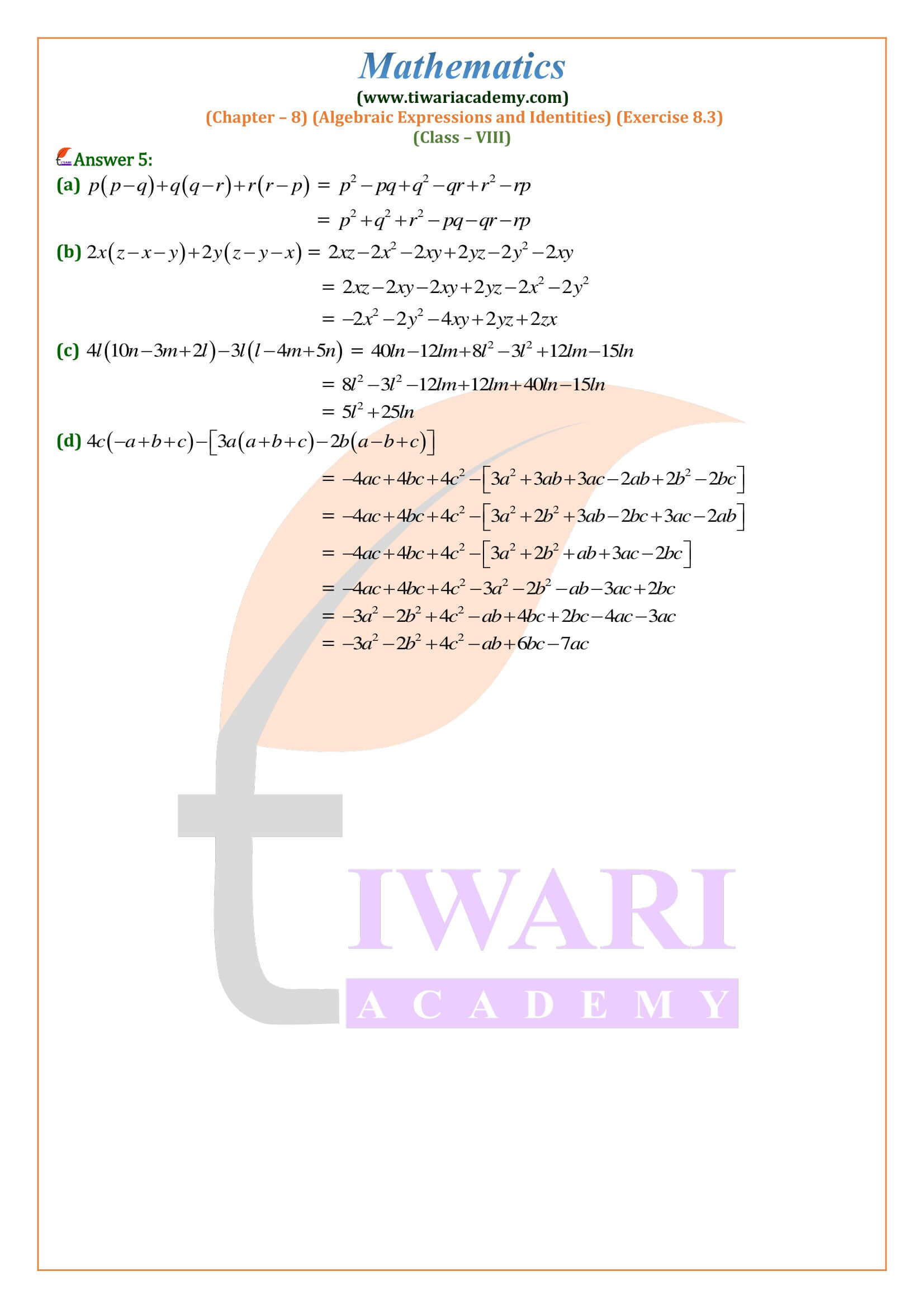
CBSE NCERT Solutions for Class 8 Maths Chapter 8 Exercise 8.3 Algebraic Expressions and Identities in Hindi and English Medium updated for 2024-25 session. The revised solution of ex. 8.3 of class 8 mathematics are given here, which is based on new textbooks issued for 2024-25 Examination.
8th Maths Exercise 8.3 Solution in Hindi and English Medium
| Class: 8 | Mathematics |
| Chapter: 8 | Exercise: 8.3 |
| Topic Name: | Algebraic Expressions and Identities |
| Content Type: | Text and Videos Format |
| Session: | CBSE 2024-25 |
| Medium: | Hindi and English Medium |
Class 8 Maths Chapter 8 Exercise 8.3 Solution
Class VIII Mathematics NCERT textbook Ex. 8.3 Algebraic Expressions and Identities in Hindi and English Medium updated for academic session 2024-25. The entire content on Tiwari Academy is free to use without any formal registration. We never call or message to user for any reason. If user need help, he can contact us for the problem. Contents in PDF, images and videos are placed on related pages in easy to use mode.

Multiplication of Algebraic Expressions
Before taking up the product of an algebraic expressions, let us look at two simple rules.
(i) The product of two factors with like signs is positive, and the product of two factors with unlike signs is negative.
(ii) If x is a variable and m, n are positive integers, then (x3 X x5) = x(3 + 5) = x8
Thus, (x6 X x4) = x(6 + 4) = x10 , etc.
Multiplication of Two Monomials
Rule: Product of two monomials = (product of their numerical coefficients) x (product of their variable parts)
Find the product of: (i) 6xy and -3x²y² (ii) 7ab², -4a²b and -5abc
We have:
(i) (6xy) x (-3x² y³) = {6 x (-3)} x {xy X x² y³}
= -18 {x³ y⁴} = -18x³ y4
(ii) (7ab²) x (–4a² b) x {–5abc} = {7 x (–4) x (–5) x {ab² x a² b x abc}
140a⁴ b⁴ c = 140a⁴ b⁴ c.
Multiplication of A Polynomial by a Monomial
Rule
Multiply each term of the polynomial by the monomial, using the distributive law:
a x (b + c) = a x b + a x c
Find each of the following products: (i) 5a² b² (3a² – 4ab + 6b²)
We have:
(i) 5a² b² (3a² – 4ab + 6b²) = (5a² b²) x (3a) + (5a² b²) x (–4ab) + (5a² b²) x (6b²)
15a³b² – 20a³b³ + 30a²b⁴
Multiplication of Two Binomials
Suppose (a + b) and (c + d) are two binomials. By using the distributive law of multiplication over addition twice, we may find their product as given below.
(a+ b) x (c + d) = a x (c + d) + b x (c + d)
= (a x c + a x d) + (b x c + b x d) = ac + ad + bc +bd.
This method is known as the horizontal method.
Multiply (3x + 5y) and (5x – 7y).
We have:
(3x + 5y) X (5x – 7y) = 3x X (5x – 7y) + 5y X (5x – 7y) = (3x X 5x – 3x X 7y) + (5y X 5x – 5y X 7y)
= (15x² – 21xy) + (25xy – 35y²) = 15x² + 4xy – 35y²
What do you learn about polynomial multiplication in exercise 8.3 of class 8 Maths?
To multiply two polynomials: Multiply each term in one polynomial by each term in the other polynomial. add those answers together, and simplify if needed.
What are the properties of polynomial multiplication given in class 8 Maths exercise 8.3?
Distributive property
When multiplying polynomials, the distributive property allows us to multiply each term of the first polynomial by each term of the second. We then add the products together and combine like terms to simplify.
Which is the most important topic in class 8 Maths exercise 8.3?
In class 8 Maths exercise 8.3 the multiplying polynomials is very important. Multiplying polynomials involves applying the rules of exponents and the distributive property to simplify the product. Polynomial multiplication can be useful in modeling real world situations. Understanding polynomial products is an important step in learning to solve algebraic equations involving polynomials.