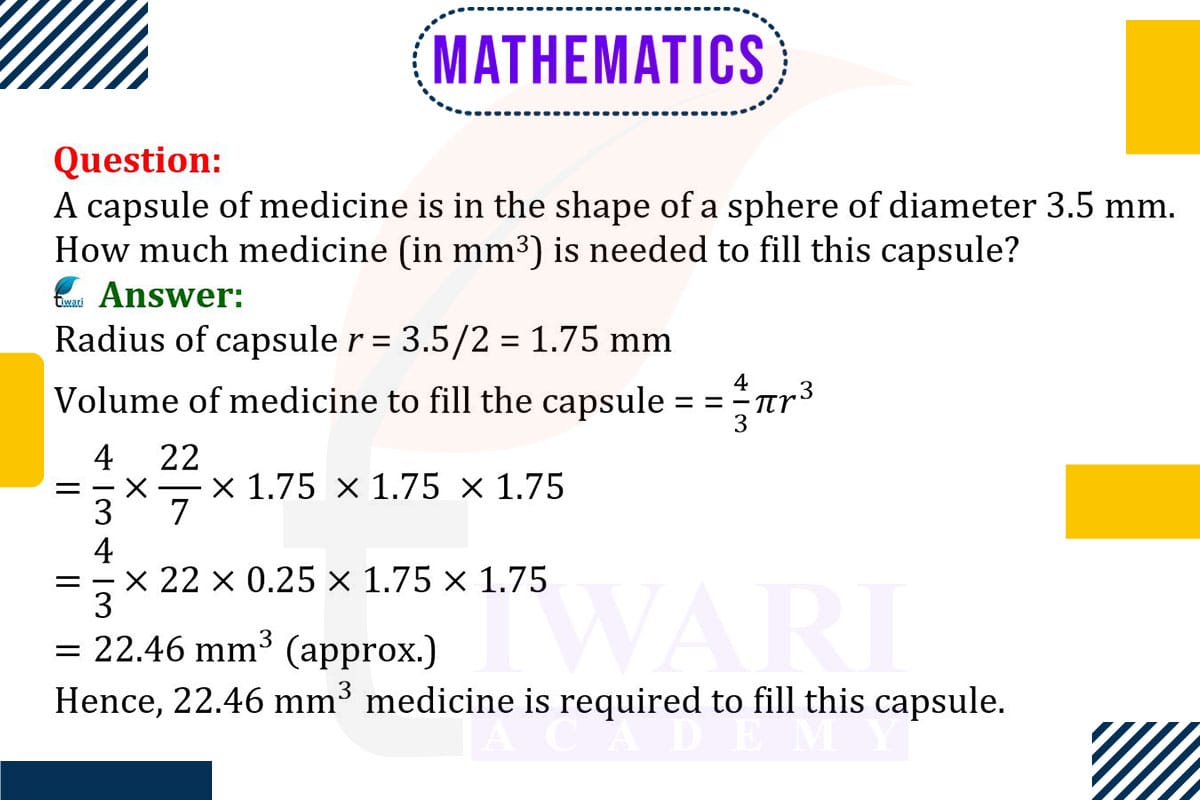
To find the amount of medicine needed to fill a spherical capsule, we calculate the volume of the sphere. The volume of a sphere is given by the formula V = (4/3)πr³, where r is the radius of the sphere.
The diameter of the capsule is 3.5 mm, so the radius r is half of this, which is 1.75 mm. Substituting this into the volume formula, we get V = (4/3)π × 1.75³ mm³.
This calculation will give the volume of the capsule in cubic millimeters, which is the amount of medicine needed to fill it. This approach demonstrates how geometric principles are applied in practical scenarios, such as pharmaceuticals, to determine quantities and capacities.
Let’s discuss in detail
Volume Calculation in Pharmaceutical Applications
The calculation of the volume of a capsule is a critical task in pharmaceutical manufacturing, where precise dosages and measurements are paramount. A capsule, often in the shape of a sphere, requires an accurate determination of its volume to ensure the correct amount of medicinal substance is contained within. This task not only exemplifies the practical application of geometry in medicine but also highlights the importance of precision in health-related manufacturing processes.
Understanding the Geometry of a Spherical Capsule
A spherical capsule is a three-dimensional object characterized by its diameter and radius. The diameter is the straight line passing through the center of the sphere from one side to the other. In this case, the diameter of the capsule is given as 3.5 mm. The radius, which is half the diameter, is a crucial measurement in calculating the volume of the sphere. It determines the size of the capsule and, consequently, the amount of medicine it can hold.
Calculating the Radius of the Capsule
To calculate the volume of the capsule, the first step is to determine its radius. The radius r is half of the diameter. Therefore, for a capsule with a diameter of 3.5 mm, the radius is 1.75 mm. This radius is a fundamental dimension in the formula for calculating the volume of a sphere and is essential for determining the capacity of the capsule.
The Formula for Sphere Volume
The volume of a sphere is calculated using the formula V = (4/3)πr³, where V represents the volume and r is the radius. This formula is derived from the principles of integral calculus and provides a precise calculation of the space inside a spherical object. In the context of a medicinal capsule, this volume represents the amount of medicine that can be contained within.
Applying the Volume Formula to the Capsule
By substituting the radius of 1.75 mm into the volume formula, the volume of the capsule can be calculated. The calculation V = (4/3)π × 1.75³ mm³ yields the volume in cubic millimeters. This volume is the total space available inside the capsule for the medicine. Understanding this volume is crucial for pharmaceutical companies to ensure accurate dosing and packaging of the medicine.
The Role of Geometry in Pharmaceutical Manufacturing
In conclusion, the calculation of the volume of a spherical capsule is a clear example of the application of geometric principles in pharmaceutical manufacturing. This process is vital for ensuring the correct dosage of medicine is administered, reflecting the broader importance of geometry in practical and health-related fields. The ability to accurately calculate volumes and dimensions is not only a fundamental aspect of geometry but also plays a crucial role in industries where precision and accuracy are essential for health and safety.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.4 in Detail


