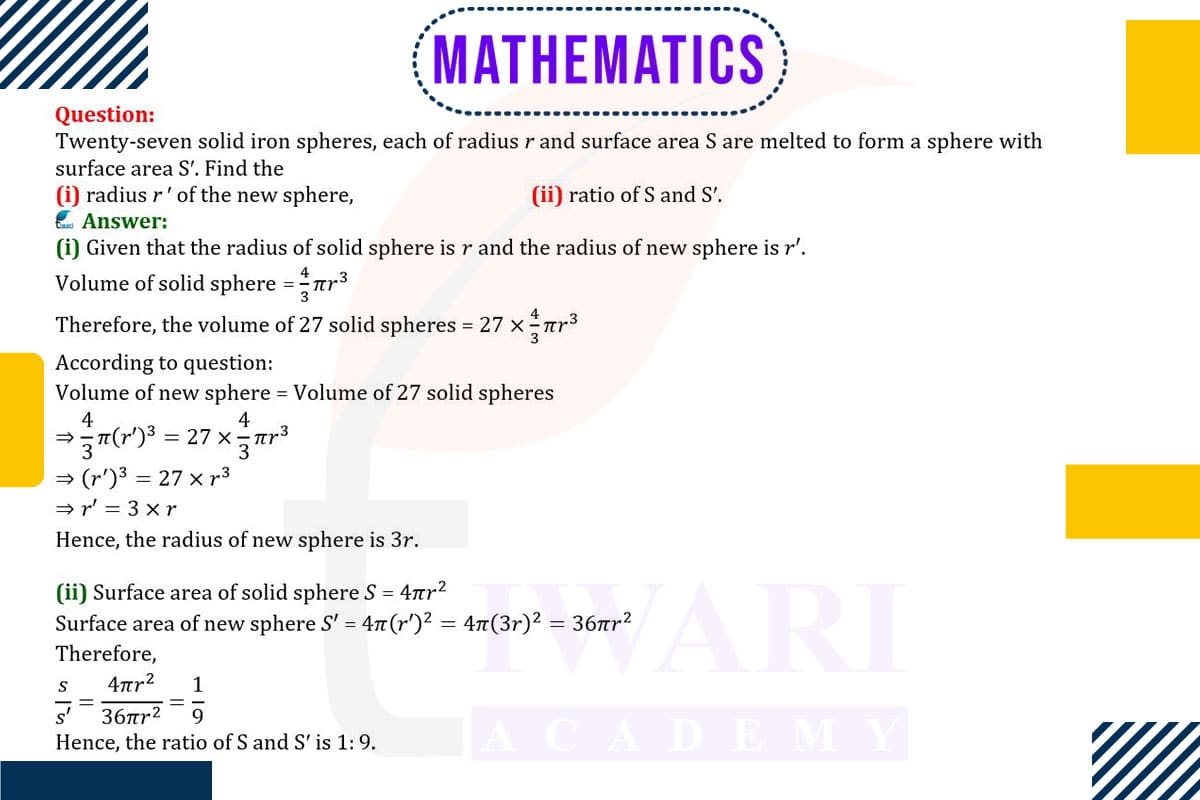
To solve this problem, we use the fact that the volume of the material remains constant during melting and reshaping.
(i) The volume of each small sphere is (4/3)πr³, and there are 27 such spheres. So, the total volume is 27 × (4/3)πr³. This total volume is equal to the volume of the large sphere formed, which is (4/3)π(r′)³. Equating these and solving for r′ = 3r.
(ii) The surface area of a sphere is 4πr². For the small sphere, it’s S = 4πr and for the large sphere, S′ = 4π(r′)². Substituting r′ = 3r into S′ and forming the ratio S/S′ gives [4πr²/(4π)(3r)²] = 1/9. Therefore, the ratio of S to S′ is 1:9. This demonstrates how properties of geometric shapes are preserved and transformed in physical processes like melting and reshaping.
Let’s discuss in detail
Volume and Surface Area in Sphere Transformation
The problem of melting and reshaping solid iron spheres into a larger sphere is a fascinating application of the principles of volume and surface area in geometry. This scenario is not just a theoretical exercise but can be encountered in manufacturing and material science. Understanding how the volume and surface area change when multiple smaller spheres are combined into a larger one provides insights into the conservation of material and the transformation of geometric properties.
Volume Conservation in Melting and Reshaping
The key principle in this problem is the conservation of volume. When the smaller spheres are melted to form a larger sphere, the total volume of the material remains constant. The volume of a sphere is given by V = (4/3)πr³, where r is the radius. For 27 smaller spheres, each with radius r, the total volume is 27 × (4/3)πr³. This total volume is equal to the volume of the new, larger sphere formed after melting.
Calculating the Radius of the New Sphere
To find the radius r′ of the new sphere, we equate the total volume of the smaller spheres to the volume of the larger sphere. The volume of the larger sphere is (4/3)π(r′)³. Setting this equal to 27 × πr³ and solving for r′ gives r′ = 3r. This calculation shows that the radius of the new sphere is three times that of the smaller spheres, illustrating how volume conservation affects dimensions in geometric transformations.
Understanding Surface Area in Geometric Transformations
The surface area of a sphere is another critical aspect of this problem. The surface area is given by S = 4πr². For the smaller spheres, each has a surface area S, and for the larger sphere, the surface area is S′ = 4π(r′)². Understanding how the surface area changes when the spheres are combined is important for applications like coating or painting, where surface area determines the amount of material needed.
Ratio of Surface Areas of the Spheres
To find the ratio of the surface areas of the original spheres to the new sphere, we use the formulae for S and S′. Substituting r′ = 3r into S′ = 4π(r′)² and forming the ratio S/S′ gives [(4πr²)/(4π(3r)²] = 1/9. This ratio of 1:9 indicates that the surface area of the new sphere is nine times that of one of the smaller spheres, highlighting a non-linear relationship between size and surface area in geometric scaling.
Implications of Geometric Principles in Material Transformation
In conclusion, this problem demonstrates the practical implications of geometric principles like volume conservation and surface area transformation in material science and manufacturing. The ability to calculate the new dimensions and surface area after a transformation is crucial in various fields, from industrial design to metallurgy. It underscores the importance of understanding geometric relationships in real-world applications, where the conservation of material and the change in surface properties play a significant role in the outcome of manufacturing and processing techniques.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.4 in Detail


