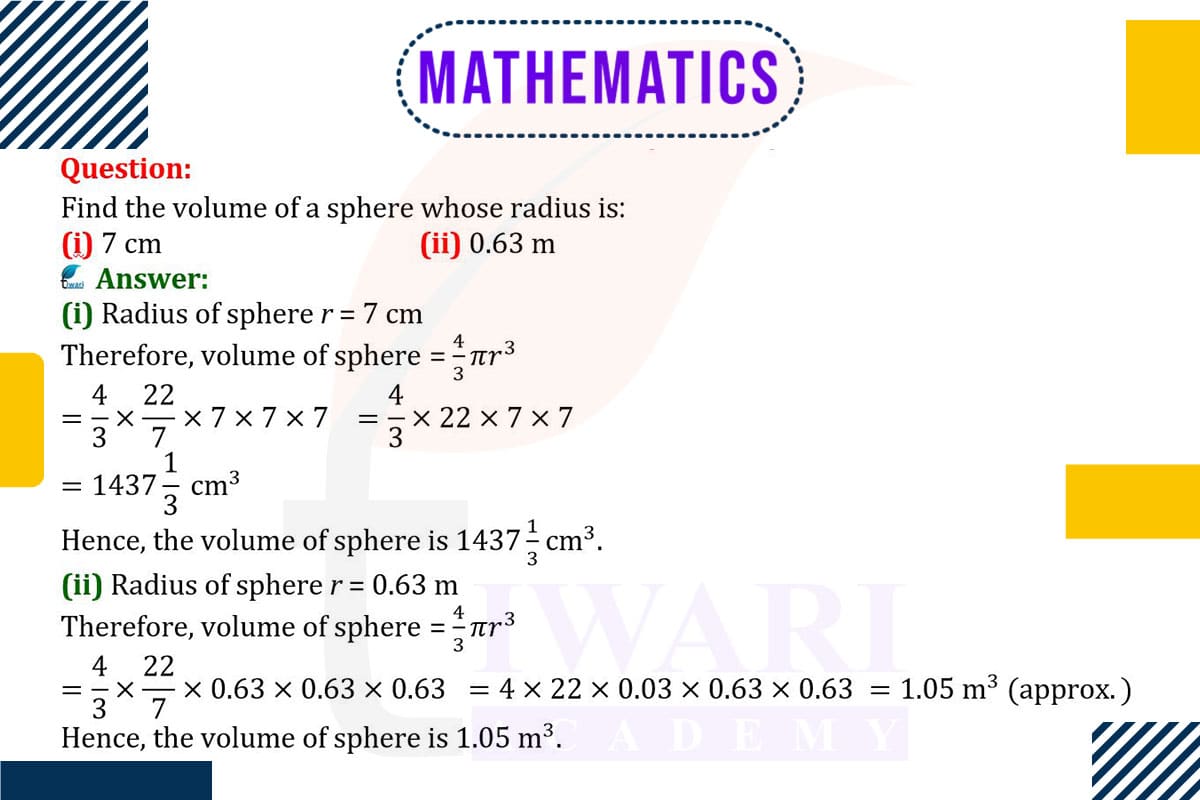
To find the volume of a sphere, use the formula V = (4/3)πr³, where V is the volume and r is the radius.
(i) For a sphere with a radius of 7 cm, substitute r = 7 cm into the formula to get V = (4/3)π × 7³ cm³.
(ii) For a sphere with a radius of 0.63 m, substitute r = 0.63 m into the formula to get V = (4/3)π × 0.63³ m³.
These calculations yield the volumes of the spheres with the given radii, demonstrating the direct relationship between the radius of a sphere and its volume.
Let’s discuss in detail
Volume Calculation of Spheres
Calculating the volume of a sphere is a fundamental concept in geometry and is essential in various fields, from engineering to environmental science. A sphere is a perfectly symmetrical three-dimensional shape, with all points on its surface equidistant from its center. The volume of a sphere is a measure of the space it occupies and is directly related to its radius. Understanding how to calculate this volume is crucial for applications that involve spherical objects, such as in designing spherical tanks, measuring the capacity of balls, or even in astronomical calculations.
The Mathematical Formula for Sphere Volume
The volume of a sphere is calculated using the formula V = (4/3)πr³, where V represents the volume and r is the radius of the sphere. This formula is derived from integral calculus but is commonly used in basic geometry. The constant π (approximately 3.14) is a fundamental aspect of the formula, reflecting the sphere’s circular cross-sections. The cube of the radius (r³) indicates that the volume of a sphere increases rapidly with an increase in its radius.
Volume Calculation for a Sphere with 7 cm Radius
For a sphere with a radius of 7 cm, the volume calculation involves substituting this value into the sphere volume formula. By placing r = 7 cm in V = (4/3)πr³, the calculation becomes V = (4/3)π × 7³ cm³. This computation will yield the volume of the sphere in cubic centimeters, providing an understanding of the amount of space it occupies. This calculation is straightforward yet crucial for accurately determining the volume of spherical objects.
Volume Calculation for a Sphere with 0.63 m Radius
Similarly, for a sphere with a radius of 0.63 meters, the volume is found by inserting r = 0.63 m into the same formula. The calculation is V = (4/3)π × 0.63³ m³. This calculation is particularly relevant in scenarios where larger spherical objects are involved, such as in architectural designs or in industrial applications. Calculating the volume in meters cubed is essential for understanding the scale and capacity of such large spherical structures or objects.
Practical Implications of Sphere Volume Calculations
The ability to calculate the volume of spheres has significant practical implications. In industrial design, it helps in creating objects with specific volume requirements. In environmental science, it aids in modeling phenomena that involve spherical shapes, like droplets. In sports, understanding the volume of balls can be crucial for design and material usage. These calculations are not just theoretical exercises but have real-world applications that impact various aspects of science, technology, and daily life.
The Importance of Geometric Volume Calculations
In conclusion, calculating the volume of spheres with different radii demonstrates the practical application of geometric principles. These calculations underscore the importance of geometry in understanding and interpreting the physical world. Whether for educational purposes, industrial applications, or scientific research, the ability to calculate and understand the volume of spherical objects is an essential skill, highlighting the relevance and utility of geometry in various fields and everyday scenarios.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.4 in Detail


