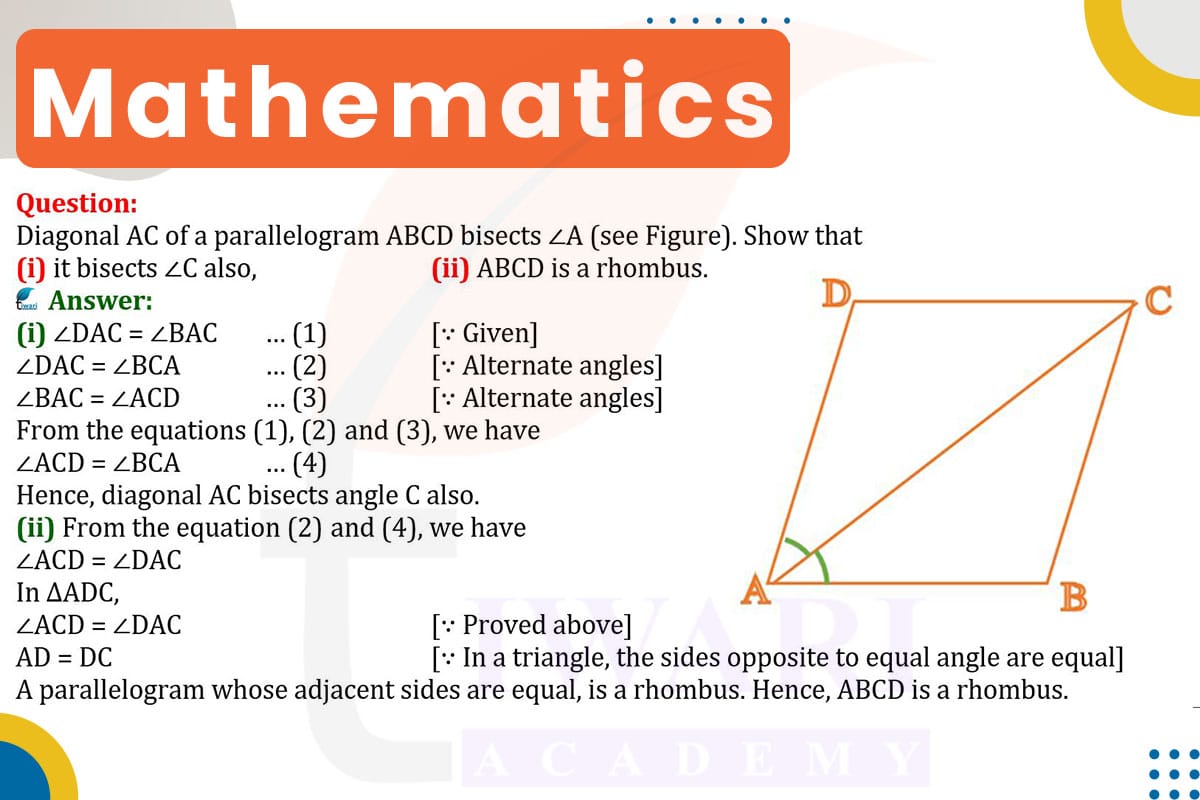
In a parallelogram ABCD, if diagonal AC bisects ∠A, then:
(i) It Bisects ∠C Also: In parallelogram ABCD, opposite angles are equal (∠A = ∠C). Since AC bisects ∠A, it divides it into two equal angles. By the properties of a parallelogram, these angles must also be equal to the angles formed by the diagonal at ∠C. Therefore, AC bisects ∠C as well.
(ii) ABCD is a Rhombus: Since AC bisects ∠A and ∠C, triangles ABC and ADC are congruent (by ASA congruence: Angle-Side-Angle). This implies AB = BC = CD = DA. Hence, ABCD is not just a parallelogram but a rhombus (all sides equal).
Let’s discuss in detail
Introduction to Parallelogram ABCD
In the study of geometry, a parallelogram is a fascinating shape with pairs of parallel and equal opposite sides. Consider parallelogram ABCD, where we are given that diagonal AC bisects ∠A. This specific condition leads to intriguing geometric properties and conclusions about the nature of parallelogram ABCD. Understanding how the properties of angles and sides in a parallelogram interact provides insight into the shape’s characteristics and helps in deducing further properties, such as whether ABCD could be a special type of parallelogram, like a rhombus.
Diagonal AC Bisects ∠A
The statement that diagonal AC bisects ∠A means that it divides ∠A into two equal angles. In other words, ∠BAC and ∠CAD are congruent. This bisecting property of the diagonal is crucial as it introduces a symmetry in the angles at vertex A. This symmetry is not a common feature in all parallelograms and suggests that ABCD might have additional special properties, setting it apart from a standard parallelogram.
Bisecting ∠C as Well
To show that diagonal AC also bisects ∠C, we utilize the fundamental properties of parallelograms. In any parallelogram, opposite angles are equal. Therefore, ∠A is equal to ∠C in parallelogram ABCD. Since AC bisects ∠A, creating two equal angles, and ∠A equals ∠C, it follows logically that the angles formed at ∠C by the diagonal AC must also be equal. Hence, diagonal AC bisects ∠C, mirroring the angle bisecting that occurs at ∠A.
Implications for Triangles ABC and ADC
The bisecting of angles ∠A and ∠C by diagonal AC has significant implications for triangles ABC and ADC. Since ∠BAC is congruent to ∠CAD (as AC bisects ∠A) and ∠BCA is congruent to ∠ACD (as AC bisects ∠C), and AC is a common side, triangles ABC and ADC are congruent by the Angle-Side-Angle (ASA) postulate. This congruence is a key element in understanding the nature of parallelogram ABCD and leads to further conclusions about its properties.
ABCD as a Rhombus
The congruence of triangles ABC and ADC implies that all sides of parallelogram ABCD are equal. This is because the congruent triangles mean that AB is equal to AD, and BC is equal to CD. Since opposite sides of a parallelogram are already equal by definition, this leads to the conclusion that all four sides of ABCD are equal. A parallelogram with all sides equal is defined as a rhombus. Therefore, parallelogram ABCD, with diagonal AC bisecting ∠A and ∠C, must be a rhombus.
The Special Nature of Parallelogram ABCD
In conclusion, the bisecting property of diagonal AC in parallelogram ABCD leads to significant geometric conclusions. By bisecting angles ∠A and ∠C, it establishes the congruence of triangles ABC and ADC, which in turn implies that all sides of the parallelogram are equal. This characteristic defines ABCD as a rhombus, a special type of parallelogram. This example illustrates the power of geometric properties and theorems in deducing the nature of shapes, showcasing the elegance and interconnectedness of geometric principles.
Discuss this question in detail or visit to Class 9 Maths Chapter 8 for all questions.
Questions of 9th Maths Exercise 8.1 in Detail


