Show that the diagonals of a square are equal and bisect each other at right angles.

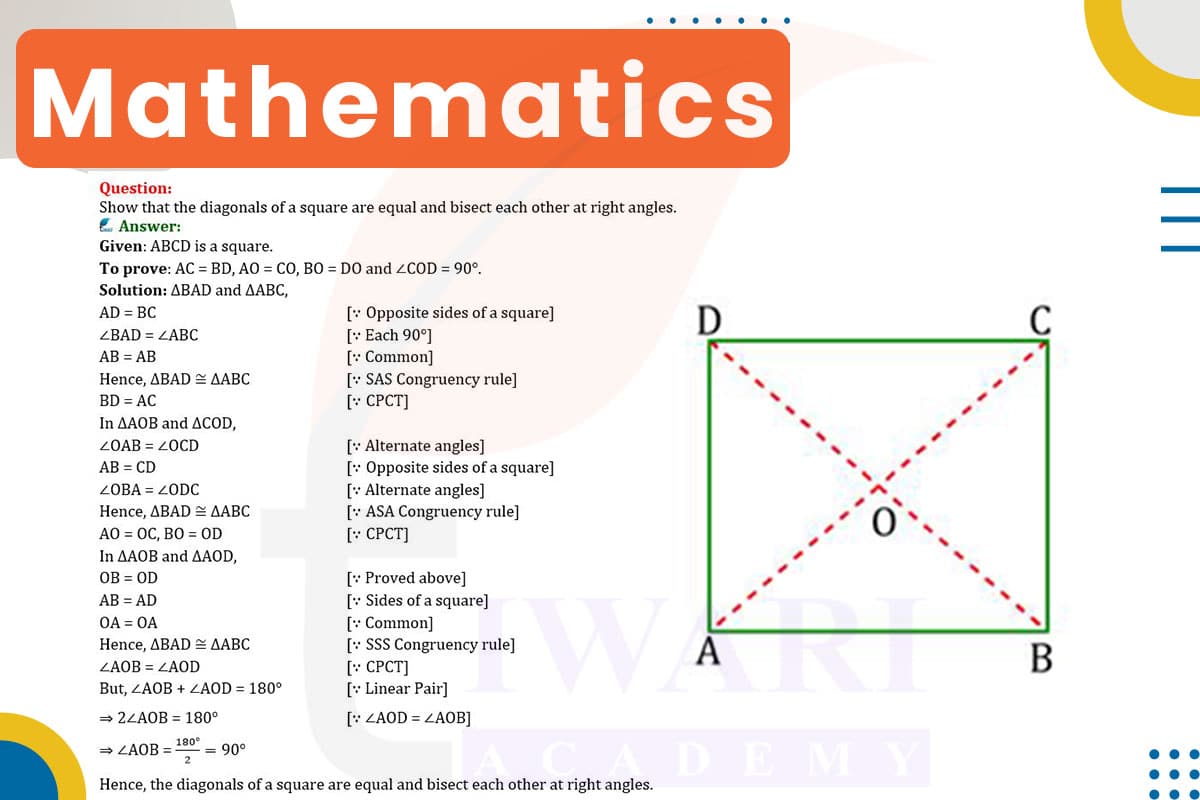
Let’s discuss in detail

A square is a fundamental shape in geometry, characterized by its four equal sides and right angles. It belongs to the family of quadrilaterals and has unique properties that distinguish it from other shapes like rectangles and rhombuses. Understanding the properties of a square, especially regarding its diagonals, is crucial in geometry. The diagonals of a square are more than just lines connecting opposite corners; they hold key geometric properties that define the nature of the square. These properties include their length, the angle at which they intersect, and how they relate to the sides of the square.

A square is defined by several key properties: all four sides are of equal length, and each of the four angles is a right angle (90 degrees). This equality of sides and angles gives the square a high degree of symmetry and regularity. Additionally, being a parallelogram, a square inherits certain properties such as opposite sides being parallel and equal in length. These inherent properties of a square set the stage for understanding the behavior of its diagonals, which are crucial in proving that they are equal and bisect each other at right angles.

In a square, the diagonals are the lines drawn from one corner to the opposite corner. These diagonals serve as a line of symmetry, dividing the square into two congruent triangles. Due to the equal length of all sides of a square, these triangles are isosceles and right-angled. The isosceles nature of these triangles implies that the diagonals are equal in length. This is because each diagonal forms the hypotenuse of two right-angled triangles, and since the sides of these triangles (which are the sides of the square) are equal, their hypotenuses (the diagonals) must also be equal.

Diagonals Bisecting at Right Angles
The diagonals of a square not only are equal in length but also bisect each other at right angles. This property comes from the fact that a square is also a rhombus (a quadrilateral with all sides equal). In a rhombus, the diagonals bisect each other at right angles. Since a square is a special case of a rhombus where all angles are right angles, this property holds true for squares as well. Therefore, when the diagonals intersect, they do so at a 90-degree angle, forming four right-angled triangles within the square.

To formally prove these properties, consider a square ABCD with diagonals AC and BD. Since ABCD is a square, AB = BC = CD = DA. Triangles ABC and ADC are isosceles with AB = BC and AD = DC. By the Pythagorean theorem, AC = BD. Since ABCD is also a rhombus, diagonals AC and BD bisect each other at right angles. Thus, at the point of intersection, which is the midpoint of both AC and BD, four right angles are formed, proving that the diagonals bisect each other at right angles.

In conclusion, the diagonals of a square are unique in that they are equal in length and bisect each other at right angles. This is a direct consequence of the square being a special type of parallelogram and rhombus. The equal length of the diagonals stems from the equal sides of the square, while the right angle at which they bisect each other is due to the square’s property as a rhombus. These properties of the diagonals are fundamental to the geometry of a square and play a crucial role in various geometric proofs and applications.

Discuss this question in detail or visit to Class 9 Maths Chapter 8 for all questions.
Questions of 9th Maths Exercise 8.1 in Detail