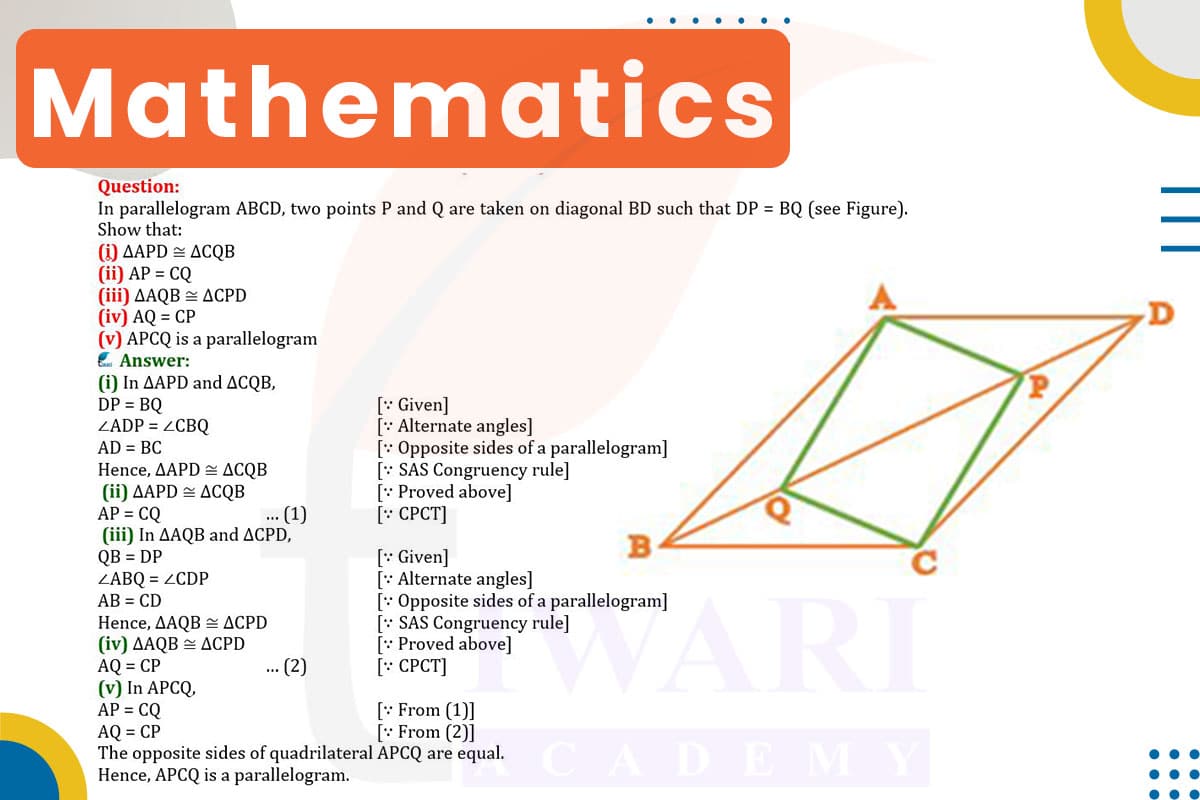
In parallelogram ABCD, two points P and Q are taken on diagonal BD such that DP = BQ. Show that: (i) Δ APD ≅ Δ CQB (ii) AP = CQ (iii) Δ AQB ≅ Δ CPD (iv) AQ = CP (v) APCQ is a parallelogram.

Let’s discuss in detail

In the parallelogram ABCD, consider two points P and Q placed on diagonal BD such that DP equals BQ. This specific placement of points P and Q on the diagonal introduces a fascinating scenario in the study of geometry. By examining the relationships and properties of the triangles formed within the parallelogram, we can uncover several congruences and equalities. These findings not only demonstrate the elegance of geometric principles but also lead to the conclusion that a new parallelogram is formed within ABCD, showcasing the depth of geometric relationships.

To prove that triangles APD and CQB are congruent, we start by observing that in parallelogram ABCD, opposite angles are equal; thus, ∠DAP (at point D) is equal to ∠BCQ (at point B). Since DP equals BQ by construction, and BD is a common side, the triangles APD and CQB have two sides and the included angle equal. According to the Side-Angle-Side (SAS) postulate of congruence in geometry, this is sufficient to establish that Δ APD is congruent to Δ CQB. This congruence is a crucial step in understanding the geometric properties of the parallelogram and the positioning of points P and Q.

From the congruence of triangles APD and CQB, it follows that corresponding parts of congruent triangles are equal. Therefore, the side AP in triangle APD is equal in length to side CQ in triangle CQB. This equality is significant as it establishes a symmetry in the parallelogram ABCD, specifically in how the diagonal BD interacts with the sides of the parallelogram. The equal lengths of AP and CQ are a direct result of the strategic placement of points P and Q on the diagonal and the inherent properties of parallelogram ABCD.

Demonstrating Δ AQB ≅ Δ CPD
Similarly, we can prove that triangles AQB and CPD are congruent. In parallelogram ABCD, ∠AQB (at point A) is equal to ∠CPD (at point C) due to the property of opposite angles being equal in parallelograms. Given that DP equals BQ and BD is a common side, triangles AQB and CPD also satisfy the SAS criterion for congruence. Thus, Δ AQB is congruent to Δ CPD. This congruence further emphasizes the symmetrical nature of the geometric construction within parallelogram ABCD.

Following the congruence of triangles AQB and CPD, it is evident that corresponding sides AQ and CP are equal. This equality is a direct consequence of the congruence of the triangles, which in turn stems from the equal angles at A and C and the equal lengths of DP and BQ. The equality of AQ and CP reinforces the symmetrical properties within parallelogram ABCD and highlights the impact of the diagonal BD and points P and Q on the internal structure of the parallelogram.

Finally, the equalities AP = CQ and AQ = CP, established through the congruences of triangles APD with CQB and AQB with CPD, lead to the conclusion that APCQ is a parallelogram. In a quadrilateral, if two pairs of opposite sides are equal, the quadrilateral is a parallelogram. Therefore, with AP equal to CQ and AQ equal to CP, APCQ satisfies the criteria for being a parallelogram. This conclusion beautifully demonstrates how specific placements of points and the application of geometric theorems can reveal hidden structures within a larger geometric figure, such as parallelogram ABCD.

Discuss this question in detail or visit to Class 9 Maths Chapter 8 for all questions.
Questions of 9th Maths Exercise 8.1 in Detail