To find the volume of wood in the pen stand, we first calculate the volume of the cuboid and then subtract the volumes of the four conical depressions.
The volume of the cuboid (the pen stand without the depressions) is given by length × width × height. So, it’s 15cm × 10cm × 3.5cm.
Each conical depression has a radius of 0.5 cm and a depth (height) of 1.4 cm. The volume of a cone is (1/3)πr²h. Therefore, the volume of one depression is (1/3)π × 0.5² × 1.4.
Since there are four such depressions, we multiply this volume by 4 and subtract it from the volume of the cuboid to get the volume of wood in the pen stand. This calculation gives us the amount of wood used in making the pen stand, accounting for the space occupied by the conical depressions.
Let’s discuss in detail
Calculating Volume in Practical Applications
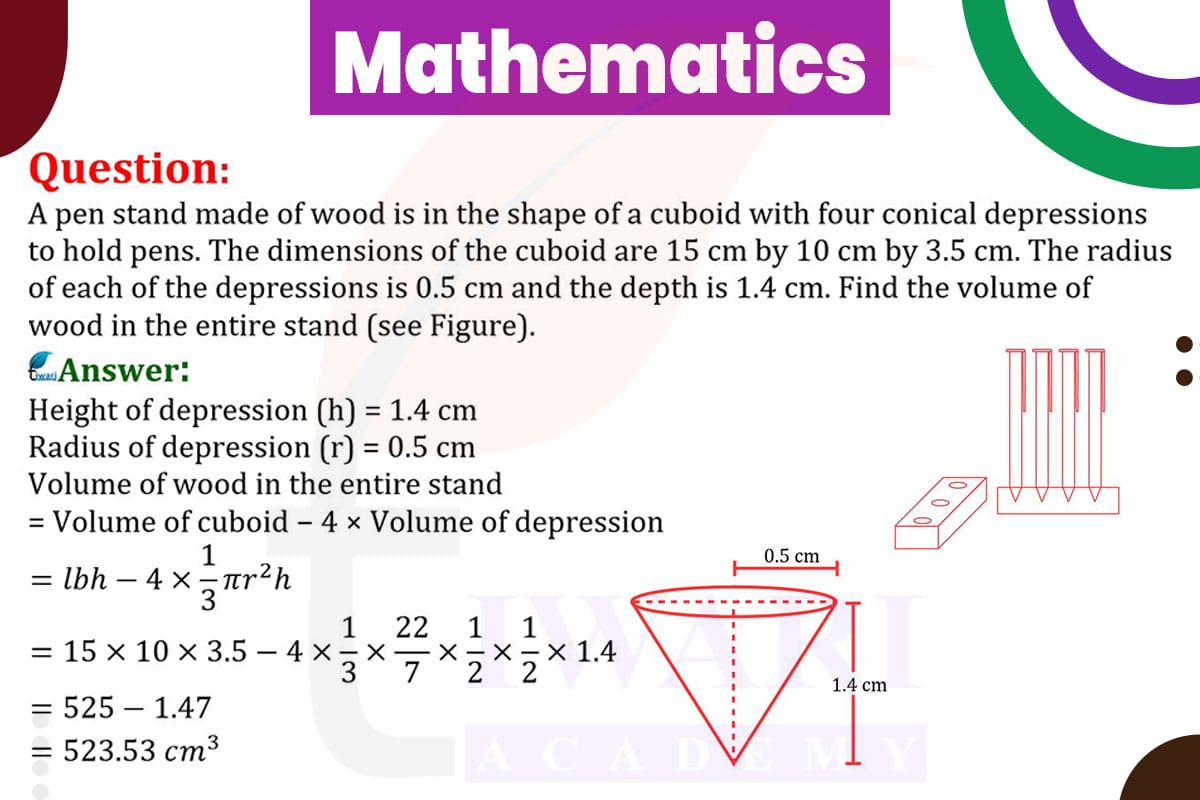
The task of calculating the volume of a wooden pen stand, which is in the shape of a cuboid with four conical depressions, is a practical application of geometry in everyday objects. This problem is not just a mathematical exercise; it’s a real-world scenario that can be encountered in fields like carpentry, design, and manufacturing. The pen stand has specific dimensions: a length of 15 cm, a width of 10 cm, and a height of 3.5 cm, with each conical depression having a radius of 0.5 cm and a depth of 1.4 cm. The challenge is to calculate the volume of wood used in the pen stand, considering the space occupied by the conical depressions.
Understanding the Cuboid Structure of the Pen Stand
The primary structure of the pen stand is a cuboid, a three-dimensional rectangle. The volume of a cuboid is calculated by multiplying its length, width, and height. In this case, the dimensions of the cuboid are 15 cm (length), 10 cm (width), and 3.5 cm (height). This straightforward calculation forms the basis for understanding the total volume of the pen stand before accounting for the conical depressions. The volume of the cuboid represents the maximum amount of wood that could be used in the pen stand.
Analyzing the Conical Depressions
The pen stand features four conical depressions designed to hold pens. Each depression reduces the total volume of wood in the stand. To calculate the volume of each depression, we use the formula for the volume of a cone, which is (1/3)πr²h, where r is the radius and h is the height (or depth) of the cone. The radius of each depression is 0.5 cm, and the depth is 1.4 cm. This calculation is crucial to determine the amount of wood removed from the cuboid to create each depression.
Calculating the Volume of the Conical Depressions
To find the total volume of the conical depressions, we calculate the volume of one cone and then multiply it by four, as there are four depressions in the pen stand. The volume of one conical depression is (1/3)π×0.5²×1.4. Multiplying this by four gives the total volume of wood removed to create the depressions. This step is essential to accurately determine the volume of wood that is actually present in the pen stand.
Total Volume of Wood in the Pen Stand
The final step is to calculate the total volume of wood in the pen stand. This is done by subtracting the total volume of the conical depressions from the volume of the cuboid. By doing this, we account for the space occupied by the depressions and thus determine the actual volume of wood used in the pen stand. This calculation is important for practical purposes such as material estimation, cost calculation, and design efficiency in woodworking and manufacturing.
The Importance of Geometry in Everyday Objects
In conclusion, calculating the volume of wood in a pen stand with conical depressions exemplifies the practical application of geometric principles in everyday objects. This exercise not only reinforces the understanding of how to calculate the volumes of composite shapes but also highlights the importance of these calculations in real-world applications, particularly in design and manufacturing. It showcases how mathematical concepts are applied in practical scenarios, emphasizing the role of geometry in the design and creation of functional and aesthetic objects. This problem-solving approach is not just about numbers and formulas; it’s about understanding the spatial properties of objects and their applications in the real world.
Discuss this question in detail or visit to Class 10 Maths Chapter 12 for all questions.
Questions of 10th Maths Exercise 12.2 in Detail


