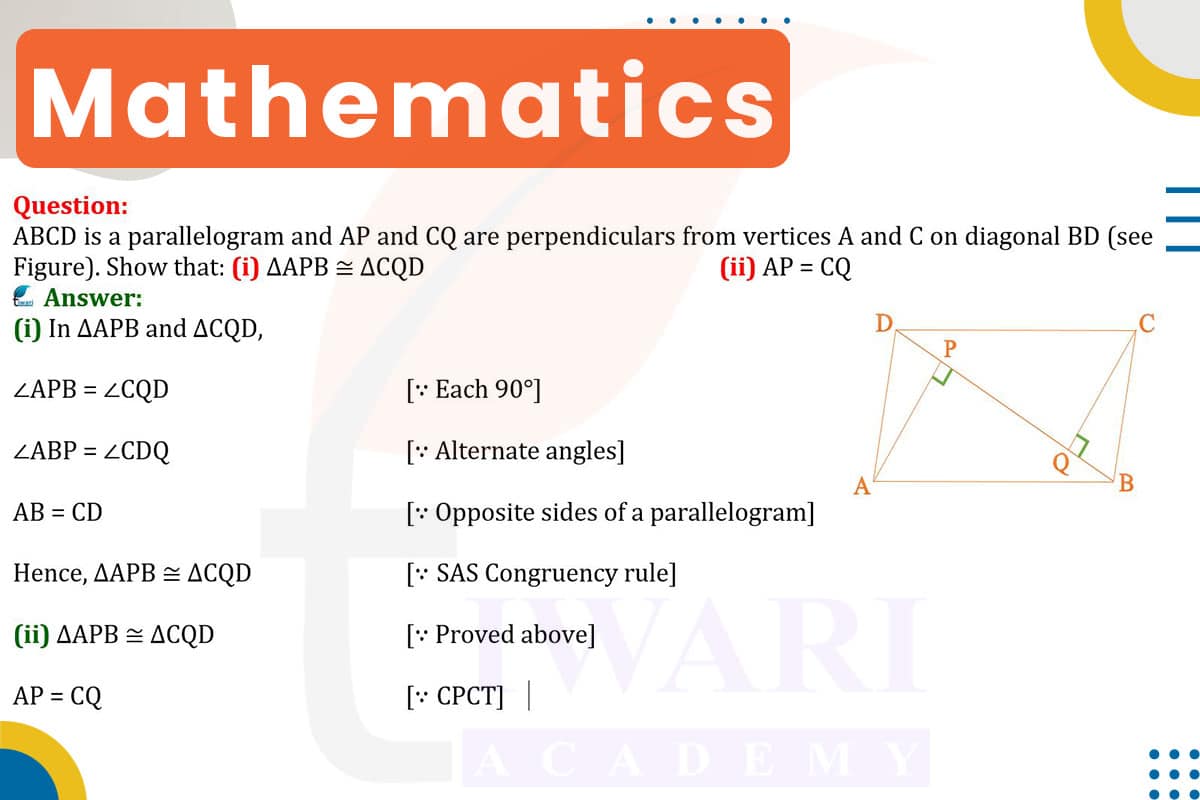
In parallelogram ABCD, with AP and CQ as perpendiculars from vertices A and C on diagonal BD:
(i) Δ APB ≅ Δ CQD: In parallelogram ABCD, opposite angles are equal, so ∠ABD = ∠CDB. AP and CQ are perpendiculars, so ∠APB and ∠CQD are right angles. Since BD is a common side, triangles APB and CQD have a right angle, a congruent angle, and a shared side (hypotenuse). By the Right Angle-Hypotenuse-Angle (RHA) criterion, Δ APB is congruent to Δ CQD.
(ii) AP = CQ: From the congruence of Δ APB and Δ CQD, corresponding sides AP and CQ are equal, as they are the legs of these right-angled triangles.
Let’s discuss in detail
Parallelogram ABCD and Perpendiculars AP and CQ
In the parallelogram ABCD, consider the perpendiculars AP and CQ drawn from vertices A and C, respectively, to the diagonal BD. This setup creates an interesting geometric scenario where the properties of parallelograms intersect with the principles of perpendicular lines and triangles. The perpendiculars introduce right angles into the structure, leading to the formation of right-angled triangles. These triangles, formed within the confines of the parallelogram, hold the key to uncovering deeper geometric relationships and congruences, which can reveal much about the nature and dimensions of ABCD.
Properties of Parallelogram ABCD
Parallelogram ABCD, by definition, has opposite sides that are equal and parallel, and opposite angles that are equal. These properties are fundamental to understanding the behavior of lines and angles within the parallelogram. When additional elements like perpendiculars from vertices to a diagonal are introduced, they interact with the parallelogram’s inherent properties to form new geometric shapes – in this case, right-angled triangles. The relationships between these triangles and the parallelogram’s sides and angles are crucial in exploring the congruences and equalities that arise.
Constructing Right-Angled Triangles APB and CQD
The perpendiculars AP and CQ from vertices A and C to diagonal BD create two right-angled triangles, APB and CQD, within parallelogram ABCD. In these triangles, AP and CQ are the perpendicular heights, and BD is the hypotenuse for both. The right angles at P and Q introduce a degree of symmetry and congruence between these triangles. This symmetry is not immediately apparent but becomes evident when considering the properties of the parallelogram and the specific nature of these right-angled triangles.
Proving Δ APB ≅ Δ CQD
To prove that triangles APB and CQD are congruent, we observe that in parallelogram ABCD, ∠ABD equals ∠CDB, as they are opposite angles. Since AP and CQ are perpendiculars to BD, ∠APB and ∠CQD are right angles. With BD as a common side (the hypotenuse of both triangles), triangles APB and CQD have a right angle, a congruent angle, and a shared side. According to the Right Angle-Hypotenuse-Angle (RHA) criterion for congruence, this is sufficient to establish that Δ APB is congruent to Δ CQD.
Establishing AP Equals CQ
From the congruence of triangles APB and CQD, it follows that corresponding parts of congruent triangles are equal. Therefore, the length of AP in triangle APB is equal to the length of CQ in triangle CQD. This equality is significant as it not only demonstrates a symmetrical relationship within the parallelogram but also reveals that the perpendicular distances from opposite vertices to the diagonal are the same. This finding is a direct consequence of the specific geometric construction within parallelogram ABCD and the properties of right-angled triangles.
Geometric Relationships in Parallelogram ABCD
In conclusion, the introduction of perpendiculars AP and CQ from vertices A and C to diagonal BD in parallelogram ABCD leads to the formation of two congruent right-angled triangles, APB and CQD. The congruence of these triangles, established through geometric principles, demonstrates that AP is equal to CQ. This equality and congruence within ABCD highlight the intricate geometric relationships that can arise in seemingly simple shapes like parallelograms. It showcases the depth of geometric principles and their ability to reveal hidden symmetries and properties in geometric figures.
Discuss this question in detail or visit to Class 9 Maths Chapter 8 for all questions.
Questions of 9th Maths Exercise 8.1 in Detail

