Area Grazed with 5m Rope: The horse can graze in a quarter circle area when tied with a 5m rope. The area is (1/4)×π×r², where r = 5 m. So, the area is (1/4)×3.14×5² = 19.625 m².
Area Grazed with 10m Rope: With a 10m rope, the horse can graze a larger quarter circle. The area is (1/4)×π×10² = 78.5 m².
Increase in Grazing Area: The increase is 78.5 − 19.625 = 58.875 m².
We can say that the grazing area increases significantly with the length of the rope.
Let’s discuss in detail
Introduction to the Problem of Grazing Area
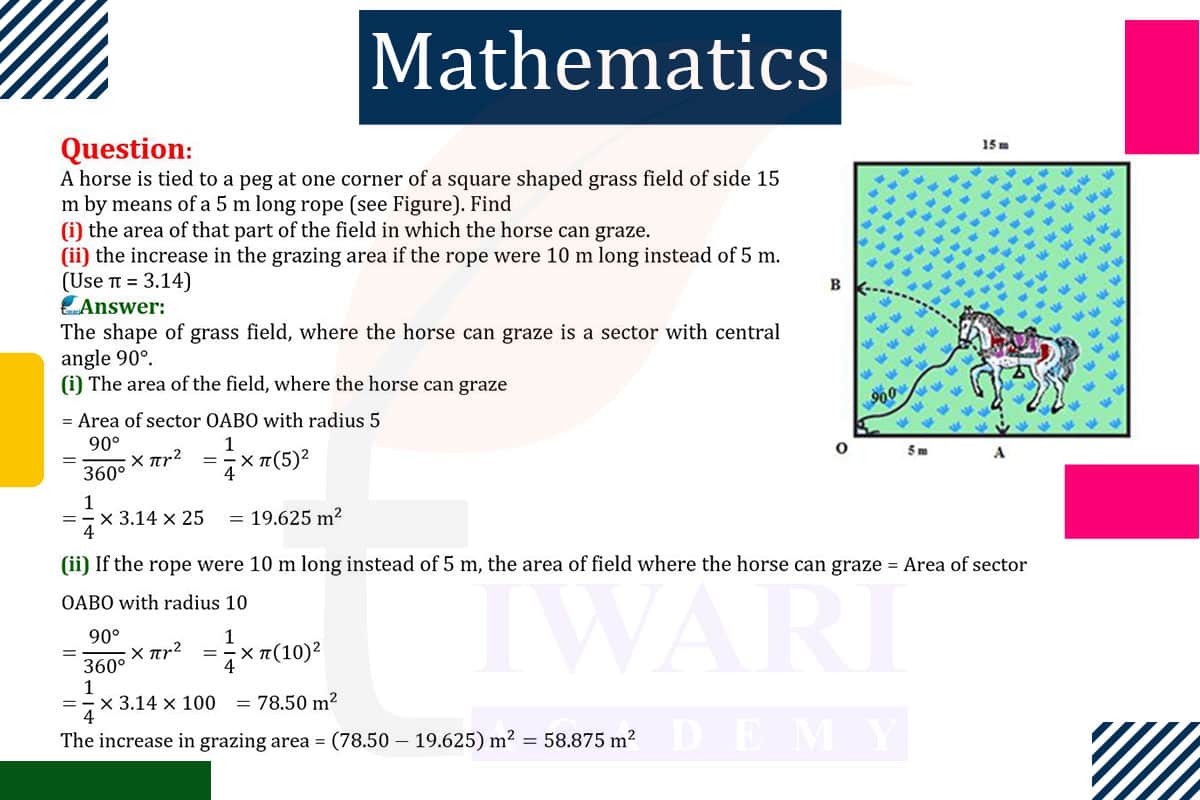
The problem at hand involves calculating the grazing area accessible to a horse tied to a peg at the corner of a square-shaped field. This scenario is a classic example of applying geometric concepts to real-world situations. The field has a side length of 15 meters, and the horse is initially tied with a 5-meter-long rope. The task is to determine the area available for grazing with this rope length and then assess how the grazing area changes if the rope’s length is increased to 10 meters. This problem not only tests our understanding of circle geometry but also illustrates how mathematical concepts can be applied to everyday situations.
Calculating the Grazing Area with a 5-Meter Rope
When the horse is tied with a 5-meter rope, the area available for grazing is a quarter of a circle, with the radius equal to the length of the rope. The formula for the area of a circle is πr², but since the horse can only access a quarter of this circle, the formula becomes (1/4)×π×r². Substituting r = 5 meters and π = 3.14, the grazing area is calculated as (1/4)×3.14×5², which equals 19.625 square meters. This represents the area in which the horse can graze when restricted by a 5-meter rope.
Understanding the Increase in Grazing Area with a Longer Rope
The problem also asks us to consider the scenario where the length of the rope is doubled to 10 meters. Intuitively, one might expect the grazing area to also double, but the relationship between the radius of a circle and its area is quadratic, not linear. This means that increasing the rope length will have a more significant impact on the grazing area than a simple doubling. The new grazing area must be recalculated using the same quarter-circle formula but with the updated radius of 10 meters.
Calculating the Grazing Area with a 10-Meter Rope
With the rope length increased to 10 meters, the grazing area becomes a larger quarter circle. Using the formula (1/4)×π×r² with r = 10 meters, the new grazing area is calculated. Substituting these values gives us (1/4)×3.14×10², which equals 78.5 square meters. This significantly larger area demonstrates how a change in the radius of a circle dramatically affects its area, a fundamental concept in circle geometry.
Analyzing the Increase in Grazing Area
To find the increase in the grazing area due to the extension of the rope, we subtract the initial grazing area (with the 5-meter rope) from the new grazing area (with the 10-meter rope). The increase is therefore 78.5 − 19.625 = 58.875 square meters. This substantial increase highlights the quadratic relationship between the radius of a circle and its area. It shows that even a small increase in the radius (or in this case, the length of the rope) can lead to a significant increase in area.
Geometric Principles in Everyday Life
In conclusion, this problem illustrates the practical application of geometric principles in everyday life. By understanding the relationship between the radius of a circle and its area, we can solve real-world problems such as determining the grazing area for a horse. This example not only reinforces the importance of geometry in practical scenarios but also demonstrates how a seemingly simple change (like increasing the length of a rope) can have a significant impact on the outcome. It’s a testament to the power and relevance of mathematics in understanding and solving everyday challenges.
Discuss this question in detail or visit to Class 10 Maths Chapter 11 for all questions.
Questions of 10th Maths Exercise 11.1 in Detail


