To find the surface area of the resulting cuboid formed by joining two cubes each of volume 64 cm³ end to end, we first determine the dimensions of each cube. The volume of a cube is given by V = a³, where a is the length of each side of the cube. Given that the volume of each cube is 64 cm³, we find the side length by solving
64 = a³, which gives a = 4 cm.
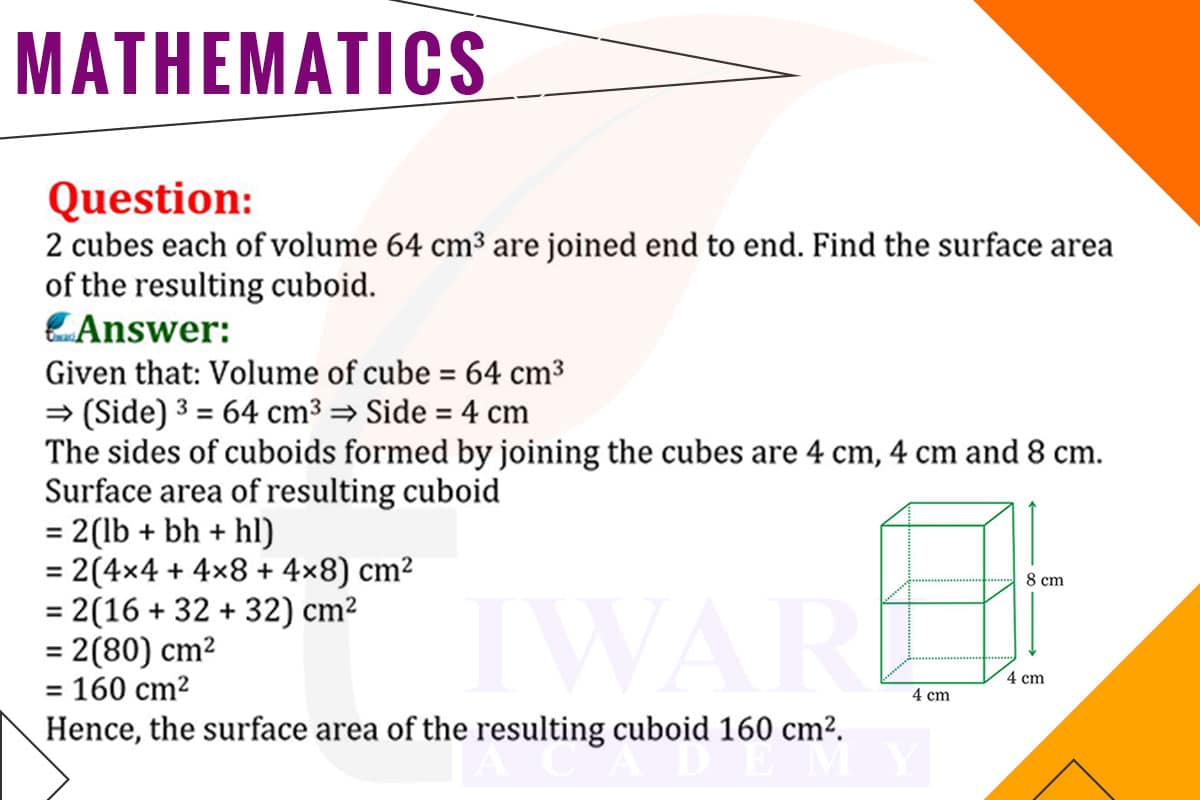
When two cubes of side 4 cm are joined end to end, the resulting cuboid will have dimensions 4 cm x 4 cm x 8 cm (since the length is the sum of the lengths of the two cubes, and the width and height remain the same as those of each cube).
The surface area SA of a cuboid is given by SA = 2(lw+lh+wh), where
l, w, and h are the length, width, and height of the cuboid, respectively. Substituting the dimensions of the cuboid, we get SA = 2(4×4+4×8+4×8) = 2(16+32+32) = 2×80 = 160 cm². Therefore, the surface area of the resulting cuboid is 160 cm².
Let’s discuss in detail
Cuboid Formation from Cubes
When we delve into the realm of geometry, particularly in the creation of complex shapes from simpler ones, we encounter intriguing scenarios. One such case is the formation of a cuboid by joining two cubes end to end. This process not only exemplifies the beauty of geometric transformations but also provides a practical application of mathematical concepts. Each cube in our scenario has a volume of 64 cm³. To understand the surface area of the resulting cuboid, we must first comprehend the properties of the individual cubes and how they contribute to the formation of the new shape. This exploration not only enhances our understanding of spatial dimensions but also sharpens our problem-solving skills.
Understanding Cube Dimensions
The journey begins with deciphering the dimensions of each cube. Given that the volume of a cube is calculated as the cube of its side (expressed as V = a³), we can reverse-engineer this formula to find the side length of our cubes. With each cube having a volume of 64 cm³, we deduce that the side length (a) is 4 cm, as 64 = a³ simplifies to a = 4 cm. This dimension is crucial as it remains constant for the width and height of the resulting cuboid, and it forms the basis for further calculations. Understanding these dimensions is not just a mathematical exercise but also a step towards visualizing how smaller units configure to form larger structures.
Formation of the Cuboid
The formation of the cuboid from two cubes is a fascinating geometric transformation. By joining the cubes end to end, we effectively create a new shape whose length is the sum of the lengths of the two cubes, while its width and height are equal to the side of a cube. This results in a cuboid with dimensions 4 cm x 4 cm x 8 cm. This process is a perfect example of how geometry can be dynamic and transformative, changing the properties and dimensions of shapes through simple operations. It also illustrates the concept of dimensional addition, where the length of the cuboid is the cumulative result of the lengths of the individual cubes.
Calculating the Surface Area
The calculation of the surface area of the resulting cuboid is the next step. The formula for the surface area of a cuboid is SA = 2(lw+lh+wh), where l, w, and h represent the length, width, and height, respectively. Substituting the dimensions of our cuboid (4 cm x 4 cm x 8 cm) into this formula, we arrive at SA = 2(4×4+4×8+4×8) = 2(16+32+32) = 2×80 = 160 cm². This calculation not only provides the surface area but also reinforces the understanding of how geometric properties are interrelated and how changes in dimensions affect overall properties.
Implications and Applications
The implications of this exercise extend beyond mere calculation. It demonstrates how basic geometric shapes can be manipulated to form more complex structures, a principle that finds applications in various fields such as architecture, engineering, and design. Understanding the transformation from cubes to a cuboid and the subsequent calculation of surface area equips us with the ability to estimate materials needed for construction, packaging, and manufacturing. This example serves as a testament to the practicality of geometric principles in real-world scenarios.
The Beauty of Geometric Transformations
In conclusion, the process of forming a cuboid from two cubes and calculating its surface area is a splendid example of the elegance and utility of geometry. It showcases how simple shapes can be combined to form more complex ones and how their properties are intricately linked. This exercise not only enhances our understanding of geometric concepts but also highlights their practical applications in everyday life. It is a reminder of how mathematics is not just about numbers and formulas but also about understanding the world around us in a more structured and logical way.
Discuss this question in detail or visit to Class 10 Maths Chapter 12 for all questions.
Questions of 10th Maths Exercise 12.1 in Detail


