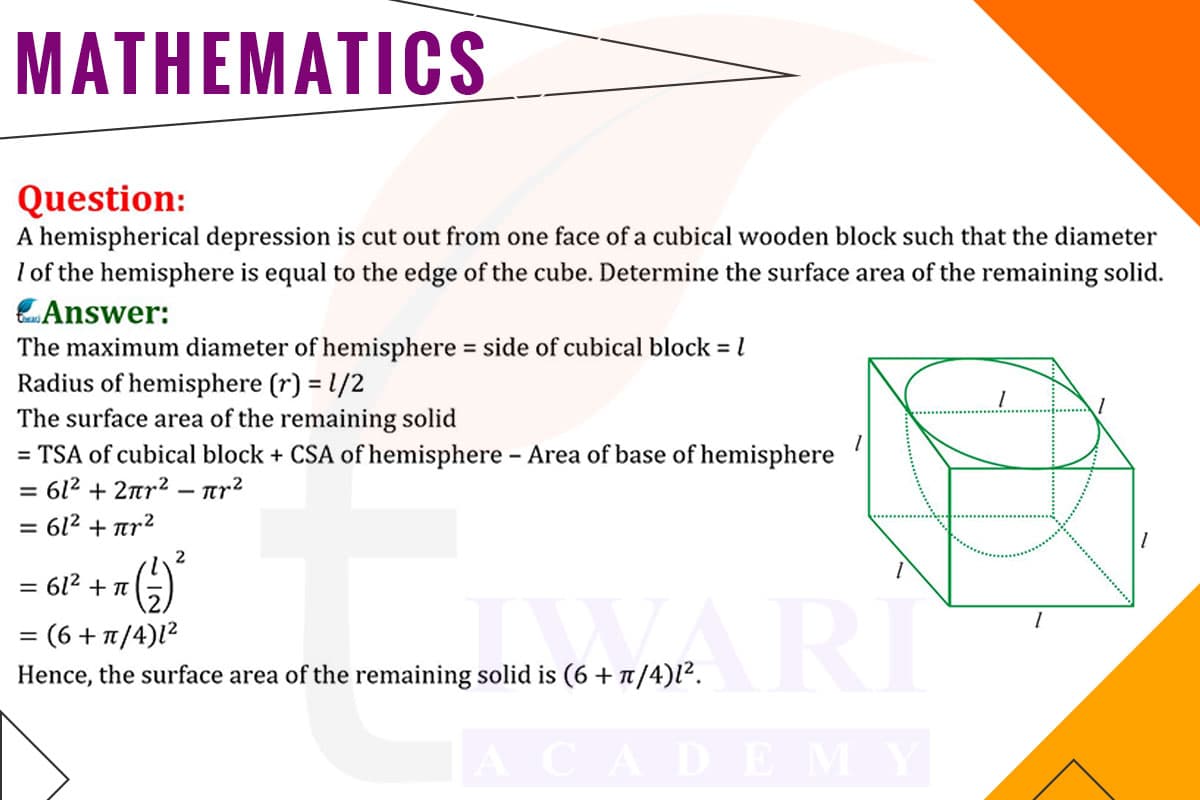
To determine the surface area of a solid formed by cutting a hemispherical depression from a cube, where the diameter of the hemisphere equals the edge of the cube, we first calculate the surface area of the cube and then subtract the area of the hemisphere that would have been exposed.
If the edge of the cube is l, the surface area of the cube is 6l². The area of the hemisphere is half the surface area of a full sphere, which is 2πr² (since the full sphere’s surface area is 4πr²). The radius r of the hemisphere is l/2.
Subtracting the hemisphere’s area from the cube’s surface area gives the surface area of the remaining solid: 6l² − 2π(l/2). This formula provides the total surface area of the solid after the hemispherical depression has been cut out.
Let’s discuss in detail
Introduction to Geometric Problem Solving
In geometry, creating new shapes by altering existing ones is a common and intriguing practice. A prime example of this is cutting a hemispherical depression from a cubical wooden block, where the diameter of the hemisphere is equal to the edge of the cube. This problem is not just about applying formulas; it’s about understanding the transformation of shapes and the resulting changes in surface area. The challenge here is to determine the surface area of the remaining solid after the hemispherical cut. This task requires a clear understanding of the properties of both the cube and the hemisphere and how they interact when part of one is removed from the other.
Understanding the Cube’s Properties
The first step in our analysis is to understand the cube’s properties. In this scenario, the cube has an edge length of l. The surface area of a cube is calculated by the formula 6l², as a cube has six faces, each with an area of l². This calculation will give us the total surface area of the cube before any modifications. It’s important to note that this surface area represents the entire outer boundary of the cube, which will be altered by the hemispherical cut.
Analyzing the Hemispherical Cut
Next, we focus on the hemispherical depression cut out from the cube. The diameter of this hemisphere is equal to the edge of the cube, which means its radius r is half the edge length, l/2. The surface area of a hemisphere is half that of a full sphere, which is
2πr² (since the surface area of a full sphere is 4πr²). This formula will be used to calculate the area of the hemisphere that is removed from the cube.
Calculating the Removed Surface Area
To find the surface area of the removed hemisphere, we substitute the radius l/2 into the hemisphere’s surface area formula. This gives us 2π(l/2), which simplifies to πl²/2. This calculation is crucial as it represents the area of the cube that is no longer part of the solid after the hemispherical cut. It’s the area that we need to subtract from the cube’s total surface area to find the surface area of the remaining solid.
Determining the Surface Area of the Remaining Solid
To determine the surface area of the remaining solid, we subtract the area of the removed hemisphere from the total surface area of the cube. The formula for this is 6l² − πl²/2. This calculation gives us the total surface area of the solid after the hemispherical depression has been cut out. It includes the surface area of the cube’s remaining faces and the flat circular area of the hemisphere that was cut out.
Synthesizing Geometric Concepts
In conclusion, calculating the surface area of a solid formed by cutting a hemispherical depression from a cube is a fascinating exercise in geometric transformation. It demonstrates how the alteration of a simple shape can lead to a more complex problem involving the combination of different geometric principles. This exercise not only reinforces our understanding of surface area calculations but also highlights the practical applications of geometry in real-world scenarios. It showcases the beauty of geometry in creating and understanding new shapes, emphasizing the importance of precise calculation and spatial reasoning.
Discuss this question in detail or visit to Class 10 Maths Chapter 12 for all questions.
Questions of 10th Maths Exercise 12.1 in Detail


