To find the angles subtended by a chord of a circle at points on the minor and major arcs, where the chord is equal to the radius of the circle, we use the properties of circles and triangles.
First, consider the triangle formed by the radius, the chord, and the line joining the center of the circle to the midpoint of the chord. This triangle is an isosceles right triangle (since the chord equals the radius), with two 45° angles at the base.
The angle subtended by the chord at a point on the minor arc is equal to the angle at the center over the chord, which is 90° (since it’s the other angle of the isosceles right triangle).
For the major arc, the angle subtended by the chord is the supplement of the angle at the center, which is 180° – 90° = 90°.
Thus, the chord subtends an angle of 90° at both a point on the minor arc and a point on the major arc.
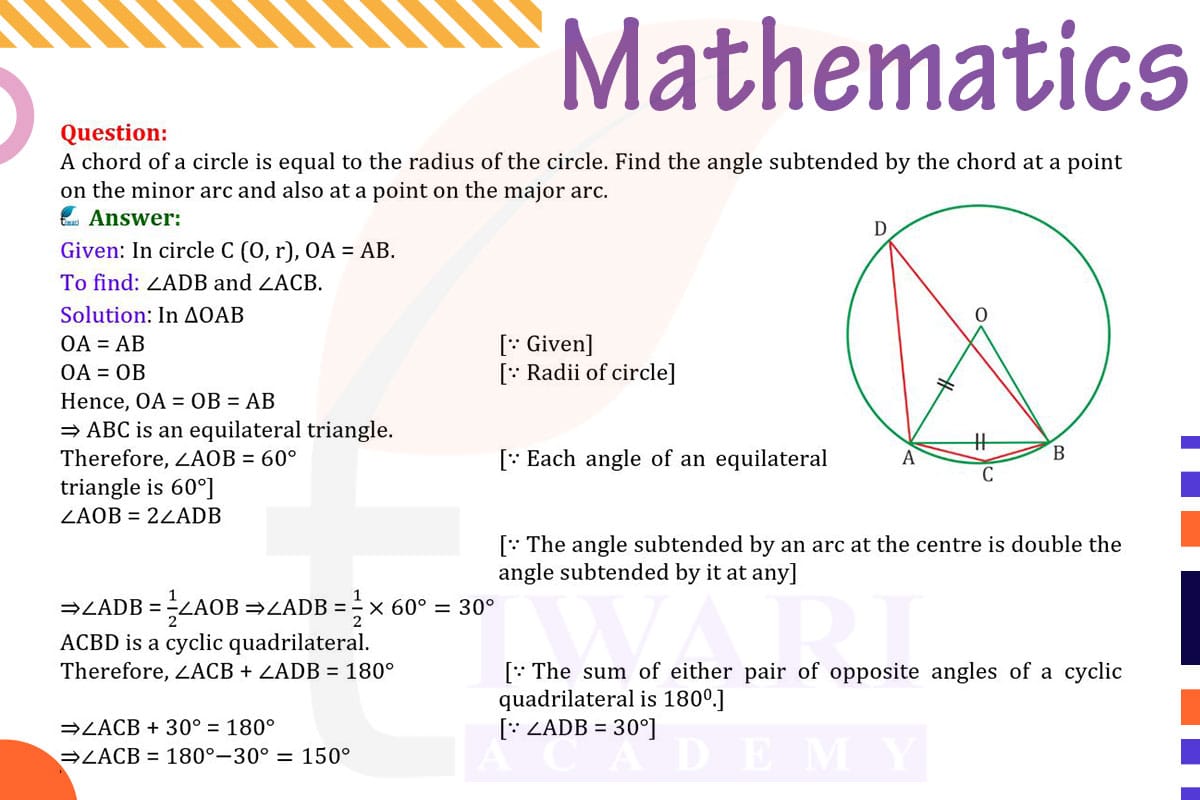
Let’s discuss in detail
Circle Geometry and Chord Properties
In circle geometry, the study of chords and the angles they subtend at various points on the circle is fundamental. A particularly interesting case arises when a chord of a circle is equal in length to the radius of the circle. This scenario sets up a unique geometric relationship within the circle, leading to intriguing questions about the angles subtended by this chord at different points on the circle, specifically on the minor and major arcs.
Understanding the Chord and Radius Relationship
In our scenario, the chord is equal in length to the radius of the circle. This equality is significant because it leads to the formation of an isosceles right triangle when we draw two radii to the endpoints of the chord. In such a triangle, the two angles at the base (adjacent to the chord) are equal, and since the sum of angles in a triangle is 180°, each of these angles is 45°.
Formation of an Isosceles Right Triangle
By connecting the center of the circle to the endpoints of the chord and to the midpoint of the chord, we form an isosceles right triangle. This triangle has two 45° angles at the base and a 90° angle at the vertex, which is the center of the circle. The 90° angle is significant as it is the angle subtended by the chord at the center of the circle.
Angle Subtended by the Chord on the Minor Arc
The angle subtended by the chord at a point on the minor arc can be understood by looking at the angle at the center of the circle over the chord. Since the chord equals the radius, the angle at the center over the chord is 90°, as established by the isosceles right triangle. Therefore, the chord subtends an angle of 90° at any point on the minor arc.
Angle Subtended by the Chord on the Major Arc
For the major arc, the angle subtended by the chord is the supplement of the angle at the center over the chord. Since the angle at the center is 90°, the angle on the major arc is 180° – 90° = 90°. This means that the chord also subtends an angle of 90° at any point on the major arc, which is a fascinating aspect of circle geometry.
Symmetry in Circle Geometry
In conclusion, a chord equal in length to the radius of a circle subtends equal angles of 90° at points on both the minor and major arcs. This result highlights the symmetry and elegance inherent in circle geometry. Such properties not only provide aesthetic satisfaction but also demonstrate the consistency and predictability of geometric principles. Understanding these relationships deepens our appreciation of geometry’s role in explaining the spatial relationships in the world around us.
Discuss this question in detail or visit to Class 9 Maths Chapter 9 for all questions.
Questions of 9th Maths Exercise 9.3 in Detail


