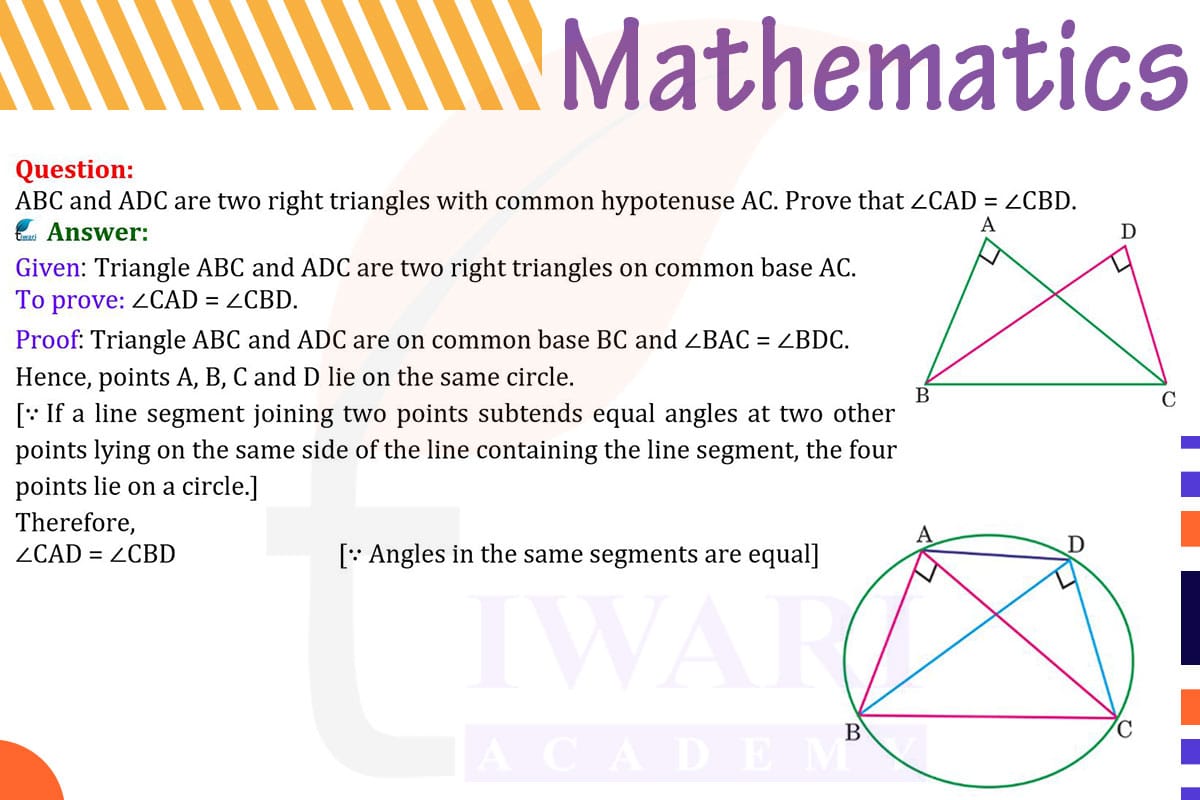
To prove that ∠CAD = ∠CBD in two right triangles ABC and ADC with a common hypotenuse AC, we use the properties of similar triangles and angles in a circle.
Since ABC and ADC are right triangles with a common hypotenuse AC, they are both inscribed in a semicircle with AC as the diameter. By the property of a circle, an angle subtended by a diameter at the circumference is a right angle. Therefore, ∠ABC and ∠ADC are both right angles.
In triangle ABC, ∠CAB + ∠ABC + ∠BCA = 180°. Since ∠ABC is a right angle, ∠CAB + 90° + ∠BCA = 180°. Thus, ∠CAB + ∠BCA = 90°.
Similarly, in triangle ADC, ∠CAD + ∠ADC + ∠ACD = 180°. With ∠ADC being a right angle, ∠CAD + 90° + ∠ACD = 180°. Hence, ∠CAD + ∠ACD = 90°.
Since ∠BCA and ∠ACD are angles at the circumference subtended by the same arc BC, they are equal. Therefore, ∠CAB + ∠BCA = ∠CAD + ∠ACD.
Given that ∠BCA = ∠ACD, it follows that ∠CAB = ∠CAD. Therefore, ∠CAD = ∠CBD, as required.
Let’s discuss in detail
Geometric Relationships in Right Triangles
In the study of geometry, the exploration of angles within right triangles, especially those sharing a common hypotenuse, reveals fascinating relationships. In this scenario, we have two right triangles, ABC and ADC, sharing a common hypotenuse AC. Our objective is to prove that ∠CAD is equal to ∠CBD. This proof involves understanding the properties of right triangles and the subtleties of angles within a circle.
The Significance of the Common Hypotenuse
The common hypotenuse AC in triangles ABC and ADC plays a crucial role. In a circle, the diameter (or hypotenuse, in this case) subtends a right angle to any point on the circle’s circumference. This means that both triangles ABC and ADC can be inscribed in a semicircle with AC as the diameter, making ∠ABC and ∠ADC right angles by definition.
Analyzing Triangle ABC
In triangle ABC, the sum of the angles must equal 180°. Given that ∠ABC is a right angle (90°), the sum of the other two angles, ∠CAB and ∠BCA, must be 90° to satisfy the angle sum property of a triangle. This relationship is key to understanding the angle measures within the triangle and their relation to the circle.
Examining Triangle ADC
Similarly, in triangle ADC, the angle sum property holds true. With ∠ADC also being a right angle (90°), the sum of ∠CAD and ∠ACD must be 90°. This parallel between the two triangles in terms of their angle sums provides a foundation for proving the equality of ∠CAD and ∠CBD.
Establishing the Equality of Angles
Since ∠BCA and ∠ACD are angles subtended by the same arc BC in the semicircle, they are equal. This equality leads to the conclusion that ∠CAB (in triangle ABC) and ∠CAD (in triangle ADC) must also be equal, as both pairs of angles add up to 90°. Therefore, ∠CAD = ∠CAB.
Proving ∠CAD Equals ∠CBD
In conclusion, through the application of the properties of right triangles and angles in a semicircle, we have demonstrated that ∠CAD is equal to ∠CBD. This proof not only highlights the elegance of geometric relationships but also underscores the importance of understanding how angles and sides interact in geometric figures, particularly in the context of circles and right triangles. Such insights are fundamental to the broader understanding of geometry and its applications.
Discuss this question in detail or visit to Class 9 Maths Chapter 9 for all questions.
Questions of 9th Maths Exercise 9.3 in Detail


