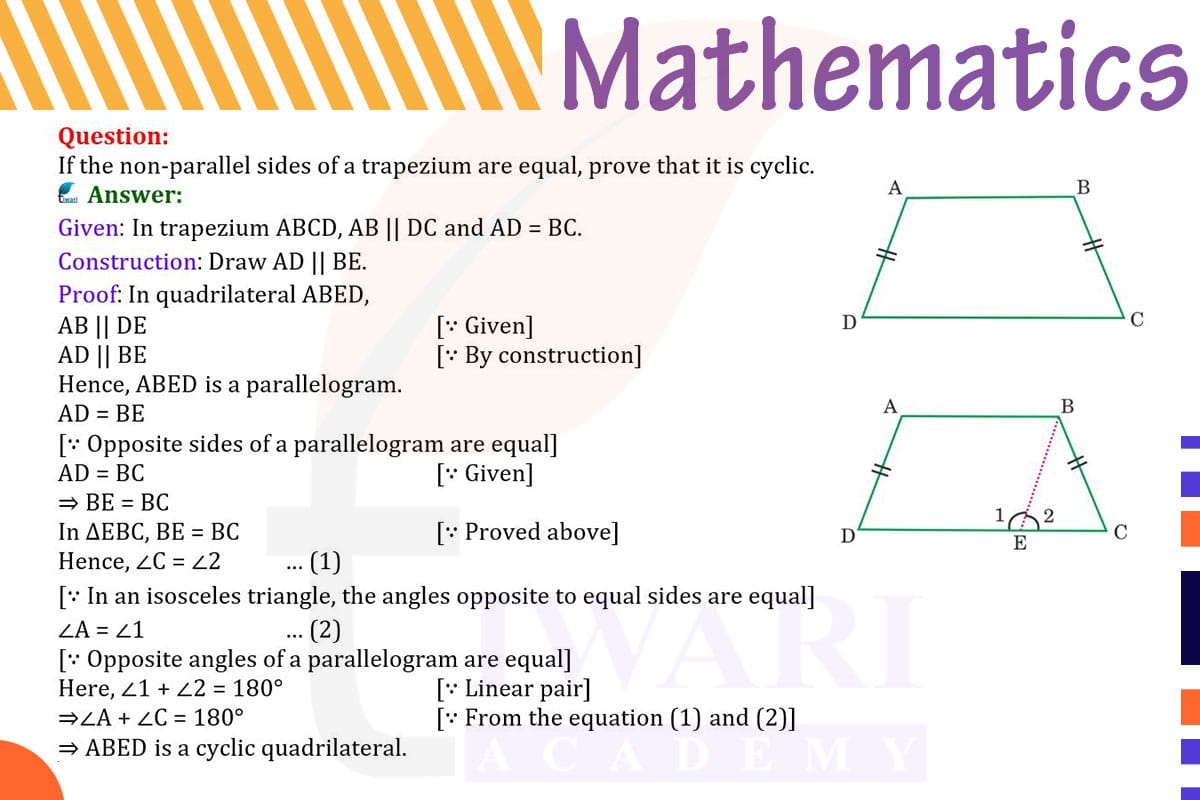
To prove that a trapezium (or trapezoid) with equal non-parallel sides is cyclic (can be inscribed in a circle), we use the property that a quadrilateral is cyclic if and only if the sum of a pair of opposite angles is 180°.
In a trapezium with equal non-parallel sides, let’s denote these sides as AB and CD. Because AB = CD, triangles ABD and BCD are isosceles. In isosceles triangles, the base angles are equal. Therefore, ∠ABD = ∠BAD and ∠BCD = ∠CDB.
Since AB and CD are non-parallel, ∠ADB and ∠BCD are supplementary (they form a linear pair). Thus, ∠BAD + ∠CDB = 180°. Similarly, ∠ABD + ∠BCD = 180°. This shows that the sum of each pair of opposite angles is 180°, proving that the trapezium is cyclic.
Let’s discuss in detail
Cyclic Quadrilaterals and Trapezium Properties
In geometry, a trapezium (or trapezoid) is a four-sided figure with at least one pair of parallel sides. A fascinating aspect of trapeziums is their potential to be cyclic, meaning all their vertices can lie on a single circle. This property becomes particularly intriguing when the non-parallel sides of the trapezium are equal in length. Our goal is to prove that such a trapezium is indeed cyclic, which involves leveraging fundamental geometric principles and properties of cyclic quadrilaterals.
Understanding the Cyclic Quadrilateral Criterion
A quadrilateral is cyclic if it can be inscribed in a circle. The key criterion for a quadrilateral to be cyclic is that the sum of each pair of its opposite angles must be 180°. This criterion forms the basis of our proof. We will demonstrate that in a trapezium with equal non-parallel sides, this condition of opposite angles summing up to 180° is met, thereby proving it to be cyclic.
Analyzing the Isosceles Triangles Formed
In our trapezium, let’s denote the equal non-parallel sides as AB and CD. The equality of these sides leads to the formation of two isosceles triangles, ABD and BCD, with AB and CD as their respective bases. In isosceles triangles, the angles opposite the equal sides are also equal. Therefore, in triangle ABD, ∠ABD = ∠BAD, and in triangle BCD, ∠BCD = ∠CDB.
Exploring Supplementary Angles in the Trapezium
The non-parallel nature of sides AB and CD implies that angles ∠ADB and ∠BCD are supplementary, as they form a linear pair. This is a crucial observation, as it sets the stage for proving the cyclic nature of the trapezium. The supplementary relationship of these angles means that their sum is 180°, which aligns with the cyclic quadrilateral criterion.
Proving the Trapezium is Cyclic
Given that ∠ABD = ∠BAD and ∠BCD = ∠CDB, and knowing that ∠ADB and ∠BCD are supplementary, we can infer that ∠BAD + ∠CDB = 180°. Similarly, since ∠ABD and ∠BCD are also supplementary, ∠ABD + ∠BCD = 180°. These equalities demonstrate that the sum of each pair of opposite angles in the trapezium is 180°, satisfying the criterion for a cyclic quadrilateral.
The Cyclic Nature of the Trapezium
In conclusion, by showing that the sum of the opposite angles in a trapezium with equal non-parallel sides is 180°, we have proven that such a trapezium is cyclic. This proof not only highlights the elegance and interconnectedness of geometric principles but also showcases the fascinating properties of specific quadrilateral configurations. Understanding these geometric relationships enriches our comprehension of shapes and their properties, illustrating the depth and beauty of geometry.
Discuss this question in detail or visit to Class 9 Maths Chapter 9 for all questions.
Questions of 9th Maths Exercise 9.3 in Detail


