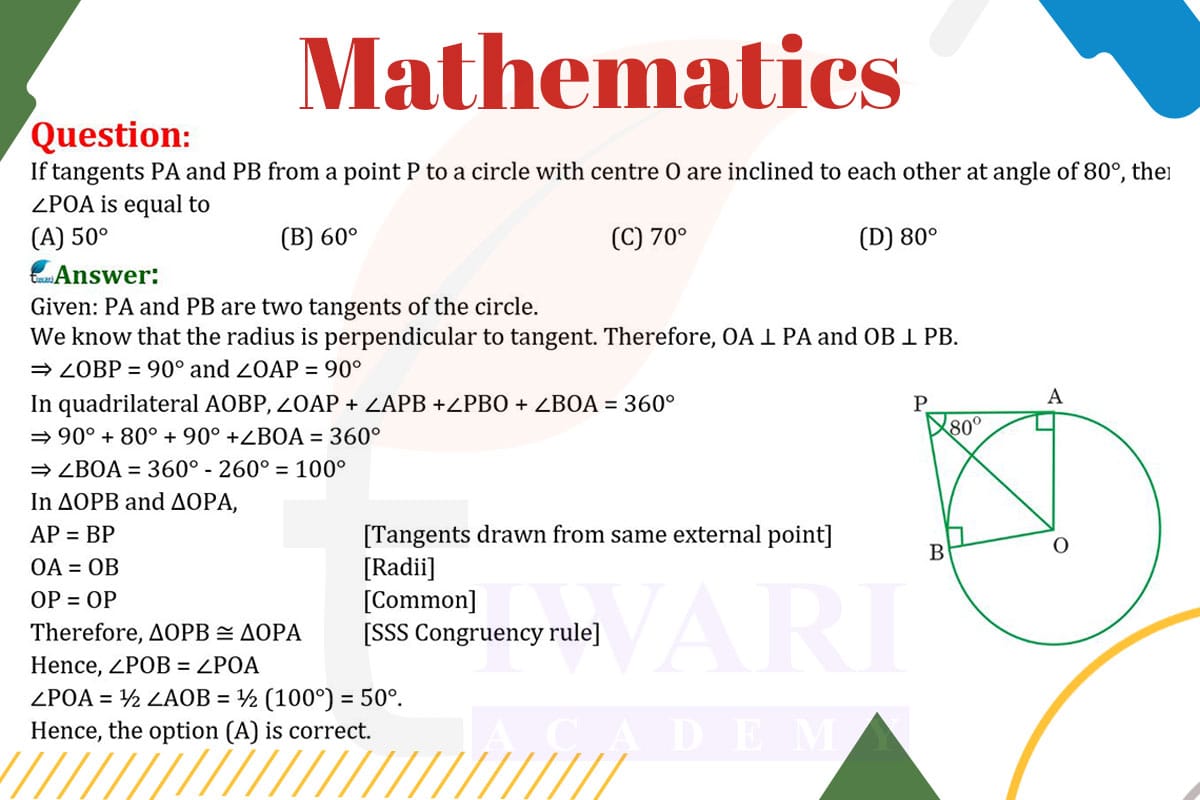
To find the angle ∠POA in a scenario where tangents PA and PB are drawn from a point P to a circle with center O, and these tangents are inclined to each other at an angle of 80°, we can use the properties of circles and tangents.
Tangents from a Point to a Circle: Tangents from a point to a circle are equal in length. Therefore, PA = PB.
Radius Perpendicular to Tangent: The radius of a circle is perpendicular to the tangent at the point of contact. Thus, ∠OPA and ∠OPB are right angles (90°).
Angle ∠APB: The angle between the two tangents PA and PB is given as 80°.
Quadrilateral OPAB: The figure OPAB is a quadrilateral with OP and OB as radii of the circle and PA and PB as tangents. The sum of the angles in any quadrilateral is 360°.
Calculating ∠POA: In quadrilateral OPAB, we have ∠OPA = 90°, ∠OPB = 90°, and ∠APB = 80°. Therefore, the sum of these three angles is 260°. Since the sum of all four angles in a quadrilateral is 360°, ∠AOB = 360° – 260° = 100°.
Hence, ∠POA is equal to 50°. This result is derived using the properties of tangents to a circle and the sum of angles in a quadrilateral.
Let’s discuss in detail
Introduction to Circle Tangent Geometry
In the fascinating world of geometry, the study of circles and their tangents presents intriguing problems that blend angles, lines, and arcs in elegant ways. A classic problem in this domain involves determining the angle formed by a radius and a tangent at the point of contact, given the angle between two tangents from a common external point to a circle. This problem not only challenges our understanding of geometric principles but also showcases the intricate relationships inherent in circle geometry.
Fundamental Properties of Tangents
A key concept in circle geometry is the nature of tangents. A tangent to a circle is a line that touches the circle at exactly one point, known as the point of tangency. When two tangents are drawn from a common external point to a circle, they are of equal length. This property is crucial in solving many geometric problems involving circles and tangents. In our specific problem, tangents PA and PB from point P to a circle with center O are inclined at an angle of 80°, setting the stage for our calculations.
The Perpendicularity of Radius to Tangent
Another important principle in circle geometry is that the radius of a circle is perpendicular to the tangent at the point of contact. This means that in our problem, the radii OA and OB are perpendicular to tangents PA and PB, respectively. This perpendicularity forms two right angles at points A and B, where the tangents touch the circle. These right angles are pivotal in determining the angle ∠POA.
The Angle Between Tangents
The angle between the two tangents PA and PB is given as 80°. This angle, ∠APB, is a critical piece of information. It helps in understanding the geometric configuration of the tangents with respect to the circle. The angle between the tangents provides a link to the angle we are trying to find, ∠POA, through the properties of the quadrilateral formed by the tangents and the radii.
Quadrilateral OPAB and Angle Sum Property
The points O, P, A, and B form a quadrilateral OPAB, with OP and OB as radii and PA and PB as tangents. In any quadrilateral, the sum of the internal angles is always 360°. Given that ∠OPA and ∠OPB are right angles (90° each) and ∠APB is 80°, we can calculate ∠POA by subtracting the sum of these three angles from 360°.
Calculating ∠POA
To find ∠POA, we add the known angles: ∠OPA (90°) + ∠OPB (90°) + ∠APB (80°) = 260°. Since the sum of angles in quadrilateral OPAB must be 360°, the remaining angle, ∠AOB, is 360° – 260° = 100°. Therefore, ∠POA is equal to 50°. This solution elegantly demonstrates how geometric principles can be applied to deduce unknown angles, highlighting the coherence and logical structure of mathematics in the context of circle geometry.
Discuss this question in detail or visit to Class 10 Maths Chapter 10 for all questions.
Questions of 10th Maths Exercise 10.2 in Detail


