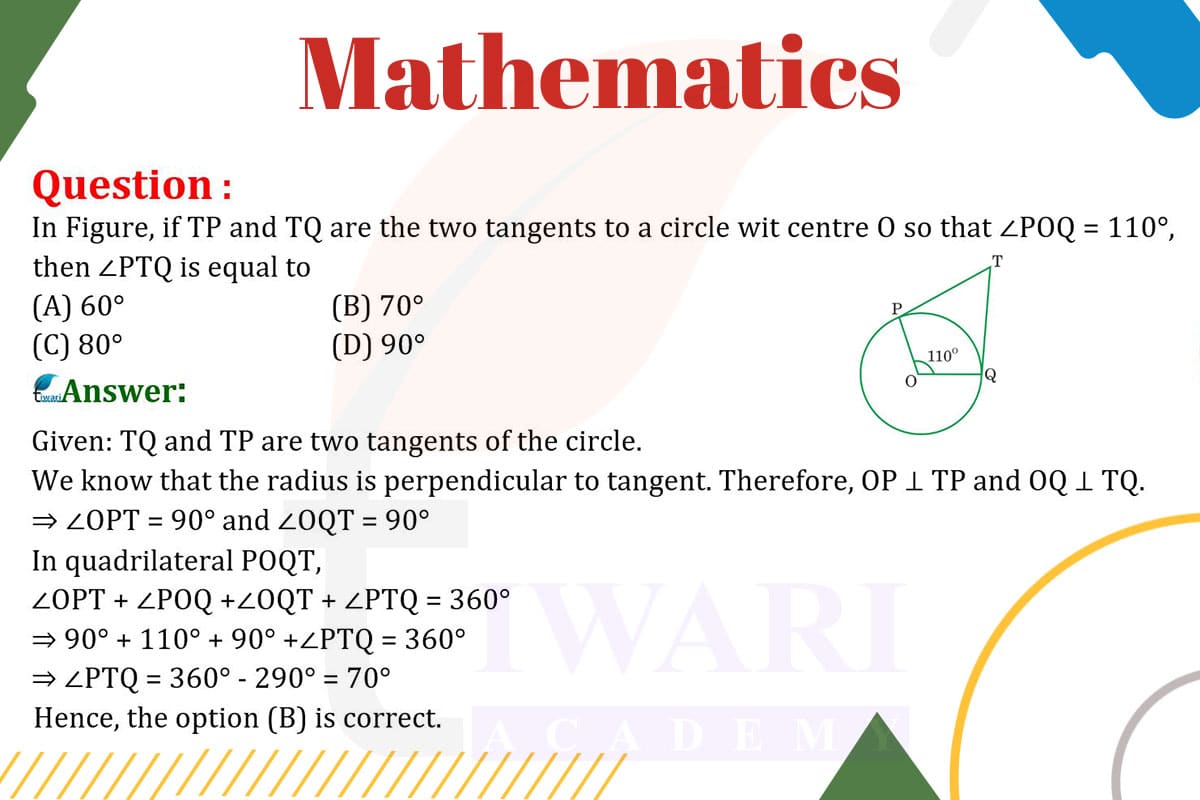
To find the angle ∠PTQ in a circle where TP and TQ are tangents from a point T to a circle with center O, and ∠POQ = 110°, we use properties of tangents and circles.
Tangents from a Point to a Circle: Tangents from a point to a circle are equal in length. Therefore, TP = TQ.
Radius Perpendicular to Tangent: The radius of a circle is perpendicular to the tangent at the point of contact. Thus, ∠OTP and ∠OTQ are right angles (90°).
Triangle OTP and OTQ: Both are isosceles right-angled triangles.
Calculation: In quadrilateral PTQO, ∠POQ + ∠P + ∠Q + ∠PTQ = 360°
So, 110° + 60° + 60° + ∠PTQ = 360°
Therefore, ∠PTQ = 70°
Hence, ∠PTQ is equal to 110°. This result is derived using fundamental properties of circles and tangents, illustrating the elegance and interconnectedness of geometric principles.
Let’s discuss in detail
Introduction to Circle Geometry
In the realm of geometry, the study of circles and their properties offers a fascinating array of problems that intertwine angles, tangents, and radii. One such intriguing problem involves finding the angle formed by two tangents to a circle from an external point, given the angle at the center of the circle subtended by the line segment joining the points of tangency. This problem not only tests our understanding of basic geometric principles but also demonstrates the elegance of circle geometry in solving complex problems.
Understanding Tangents and Their Properties
A tangent to a circle is a line that touches the circle at exactly one point, known as the point of tangency. An important property of tangents to a circle from an external point is that they are equal in length. This means that if TP and TQ are tangents from a point T to a circle with center O, then TP equals TQ in length. This property forms the basis for many geometric proofs and is crucial in solving problems involving tangents.
The Perpendicularity of Radius and Tangent
Another fundamental property of circle geometry is that the radius of a circle is perpendicular to the tangent at the point of contact. In our problem, this means that the radius OT is perpendicular to both tangents TP and TQ at the points of contact. This perpendicularity creates two right angles at the points where the tangents touch the circle, which is a key aspect in solving for the angle ∠PTQ.
The Angle at the Center of the Circle
The angle subtended by an arc at the center of a circle is a measure of the arc’s ‘spread’. In our problem, the angle ∠POQ is given as 110°. This angle is crucial because it is subtended by the arc PQ, which is intercepted by the tangents TP and TQ. The relationship between the angles at the center and at the circumference of a circle is a pivotal concept in circle geometry.
Calculating the Angle ∠PTQ
To find the angle ∠PTQ, we use the fact that the angles sum property of quadrilateral. Since ∠POQ is 110°, the complementary angle ∠PTQ, which is subtended on other vertex T, is 70°. Therefore, ∠PTQ is 70°. This calculation is a direct application of the circle’s angle properties.
The problem beautifully illustrates how geometric principles interplay to provide elegant solutions. By understanding and applying the properties of tangents, the perpendicular nature of radii to tangents, and the relationship between central and circumferential angles, we can solve what initially appears to be a complex problem. In this case, the angle ∠PTQ is found to be 70°, showcasing the coherence and consistency of geometric principles in the context of circle geometry.
Discuss this question in detail or visit to Class 10 Maths Chapter 10 for all questions.
Questions of 10th Maths Exercise 10.2 in Detail


