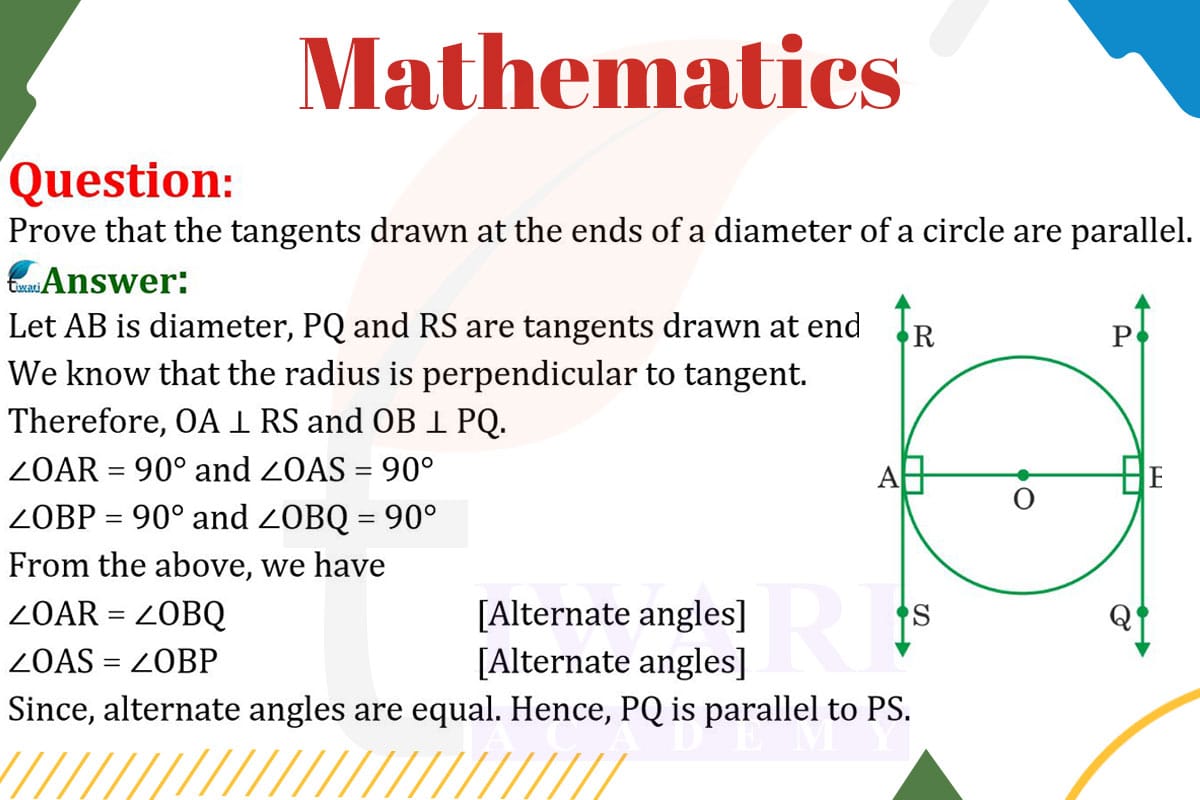
To prove that tangents drawn at the ends of a diameter of a circle are parallel, consider a circle with center O and a diameter AB. Draw tangents at points A and B, and let these tangents be line l at A and line m at B.
Radius Perpendicular to Tangent: The radius of a circle is perpendicular to the tangent at the point of contact. Therefore, OA is perpendicular to tangent l, and OB is perpendicular to tangent m.
Diameter Forms a Straight Line: The diameter AB is a straight line, meaning angle AOB is 180°.
Right Angles at Points of Tangency: Since OA is perpendicular to l, and OB is perpendicular to m, both angles OAB and OBA are right angles (90°).
Parallel Lines: If two lines are perpendicular to the same line, they are parallel to each other. Since both tangents l and m are perpendicular to the diameter AB (or its extension), they are parallel to each other.
Thus, the tangents drawn at the ends of a diameter of a circle are parallel. This conclusion is based on the fundamental properties of circles and tangents.
Let’s discuss in detail
Introduction to Circle Tangents and Diameters
In the study of circle geometry, one of the intriguing aspects is the relationship between tangents and diameters. A fundamental theorem states that tangents drawn at the ends of a diameter of a circle are parallel. This theorem not only highlights the elegant properties of circles but also plays a crucial role in various geometric constructions and proofs. Understanding this theorem requires a basic knowledge of the properties of tangents and the concept of a diameter in a circle.
Understanding Tangents to a Circle
A tangent to a circle is a straight line that touches the circle at exactly one point, known as the point of tangency. The key property of a tangent in relation to a circle is that it is perpendicular to the radius at the point of tangency. This perpendicular relationship is central to many proofs in circle geometry, including the theorem in question. It provides a foundational basis for understanding how tangents interact with other elements of a circle.
The Role of the Diameter
A diameter of a circle is a straight line that passes through the center of the circle and touches the circle at two points. It is the longest chord in a circle and bisects the circle into two equal halves. The diameter has a special property in that it subtends a right angle to any point on the circle. This property is crucial in proving the parallel nature of tangents to a circle at the ends of a diameter.
Constructing Tangents at the Ends of a Diameter
Consider a circle with center O and a diameter AB. Construct tangents at points A and B, which are the endpoints of the diameter. Let these tangents be line l at point A and line m at point B. According to the properties of tangents, line l is perpendicular to radius OA, and line m is perpendicular to radius OB. This perpendicularity is the first step in establishing the parallelism of the tangents.
Demonstrating Parallelism Through Perpendicularity
Since line l is perpendicular to OA, and line m is perpendicular to OB, and OA and OB are part of the same straight line (diameter AB), it follows that lines l and m are both perpendicular to the diameter AB. In geometry, if two lines are perpendicular to the same line, they are parallel to each other. This is because they maintain a constant angle and never meet, no matter how far they are extended.
Parallel Tangents from a Diameter
Therefore, by constructing tangents at the endpoints of a diameter and using the property that these tangents are perpendicular to the radius at the point of tangency, we can conclude that the tangents are parallel to each other. This conclusion elegantly demonstrates the interplay of basic geometric principles and the coherence of circle geometry. The theorem that tangents drawn at the ends of a diameter of a circle are parallel is a perfect example of the simplicity and beauty inherent in geometric reasoning.
Discuss this question in detail or visit to Class 10 Maths Chapter 10 for all questions.
Questions of 10th Maths Exercise 10.2 in Detail


