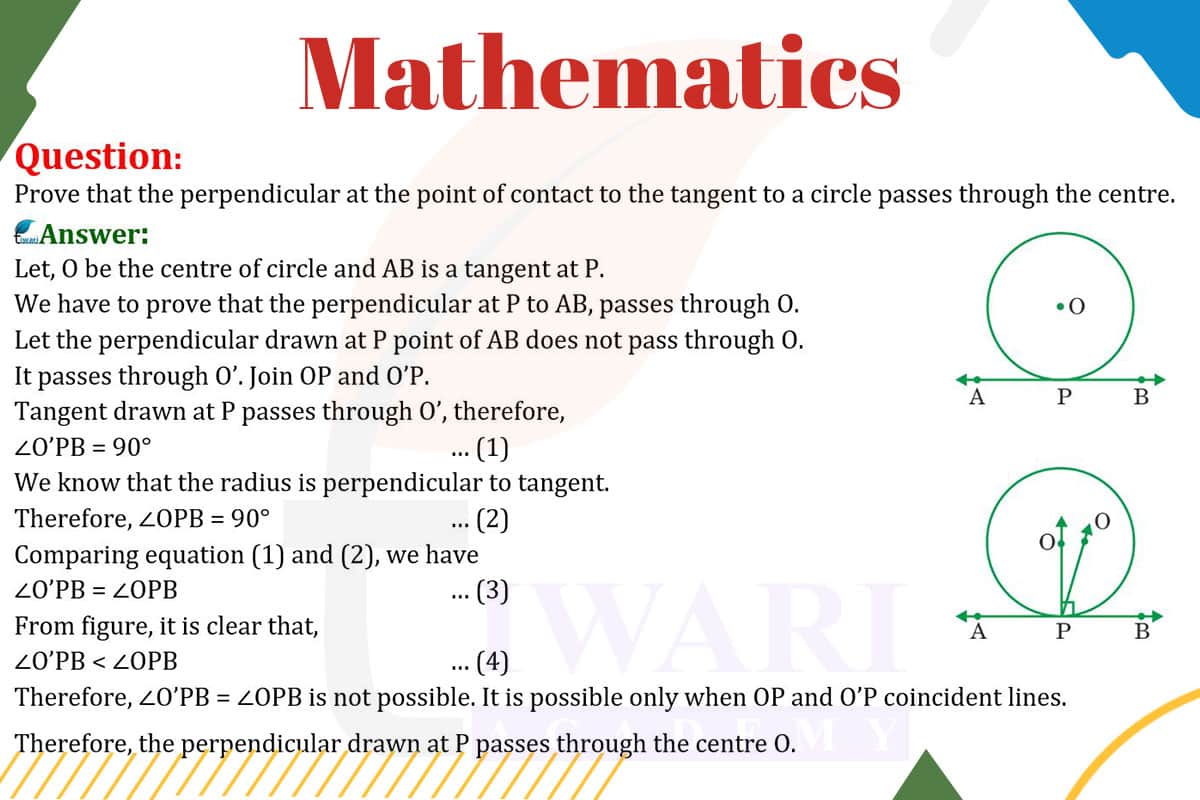
Prove that the perpendicular at the point of contact to the tangent to a circle passes through the centre.

Let’s discuss in detail

In the realm of geometry, the study of circles and their properties offers a fascinating exploration of shapes and lines. One intriguing aspect of circle geometry is the relationship between a tangent and the circle it touches. A fundamental property in this area is that the line perpendicular to the tangent at the point of contact passes through the circle’s center. This principle is not only fundamental in understanding circle geometry but also forms the basis for various geometric constructions and proofs.

A tangent to a circle is a line that touches the circle at exactly one point, known as the point of tangency. Unlike a secant, which intersects the circle at two points, a tangent only meets the circle at one point and does not cross into the circle’s interior. This unique property of tangents is key to many geometric proofs and is particularly central to the theorem that the perpendicular at the point of contact to the tangent passes through the circle’s center.

A crucial property of tangents in relation to circles is that at the point of tangency, the tangent is perpendicular to the radius of the circle. This means that if a radius is drawn from the center of the circle to the point of tangency, it forms a right angle (90 degrees) with the tangent line. This perpendicular relationship is a defining characteristic of tangents to circles and is essential for understanding their geometric properties.

Constructing the Perpendicular to the Tangent
To prove our theorem, we start by constructing a perpendicular line to the tangent at its point of contact with the circle. According to geometric principles, there can only be one unique line perpendicular to another at a given point. In this case, the constructed perpendicular line at the point of tangency must align with the radius of the circle, as it is the only line that satisfies the condition of being perpendicular to the tangent at that point.

Since the radius of a circle extends from the center to any point on the circle’s circumference, the alignment of the constructed perpendicular with the radius implies that this line must pass through the center of the circle. This is because the radius, by definition, connects the center with the circumference. Therefore, the perpendicular line we have constructed, which coincides with the radius, must also pass through the circle’s center.

Thus, we conclude that the perpendicular at the point of contact to the tangent of a circle passes through the center of the circle. This conclusion elegantly demonstrates the interplay of basic geometric principles and the coherence of circle geometry. It highlights the unique relationship between a circle, its radius, and a tangent line, showcasing the simplicity and beauty inherent in geometric reasoning. This theorem is a fundamental aspect of circle geometry and is pivotal in various geometric constructions and applications.

Discuss this question in detail or visit to Class 10 Maths Chapter 10 for all questions.
Questions of 10th Maths Exercise 10.2 in Detail