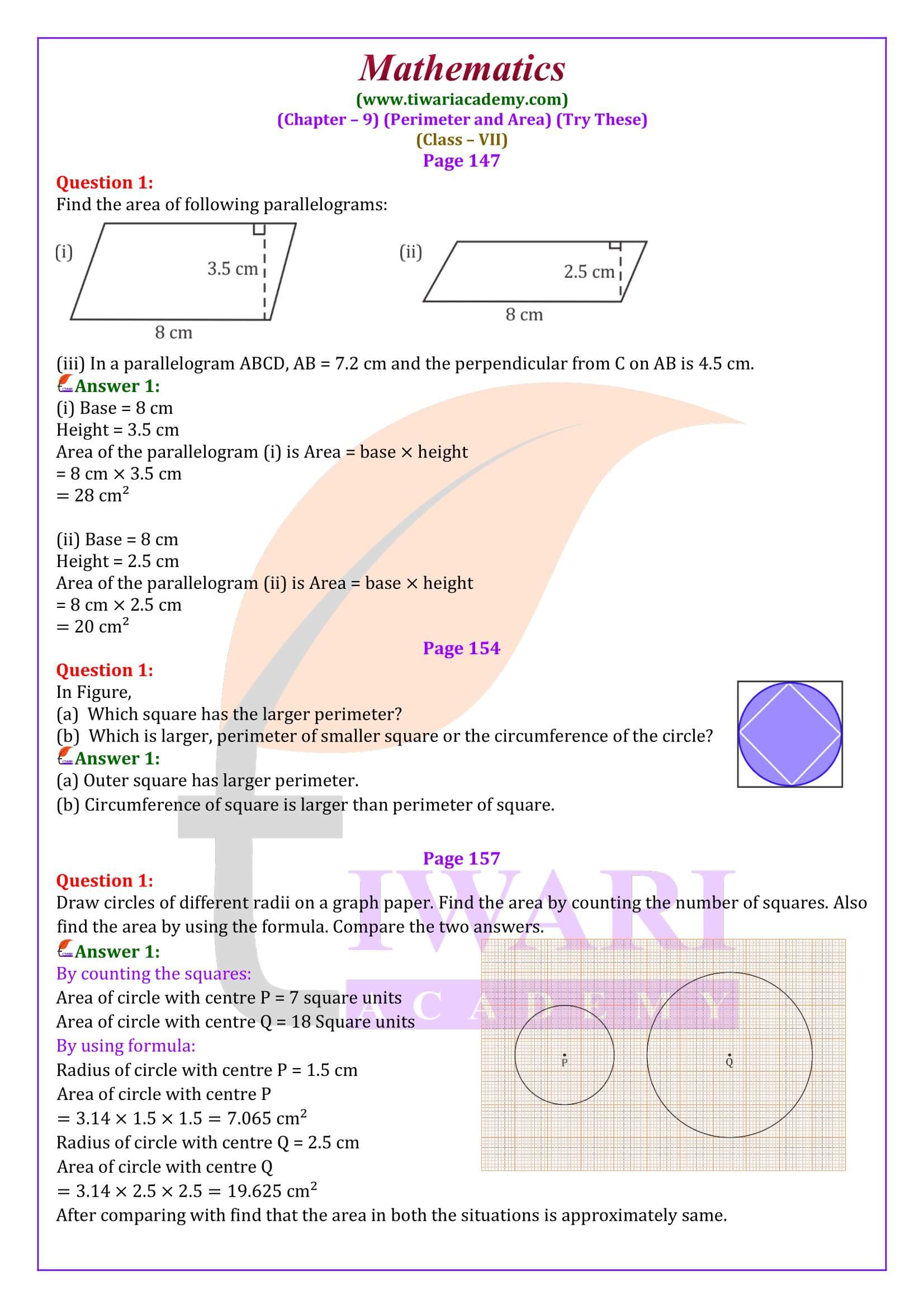
NCERT Solutions for Class 7 Maths Chapter 9 Perimeter and Area and Class 7 Maths Chapter 9 Try These Solutions in Hindi and English Medium updated for academic session 2024-25. The ease or difficulty of solving the solutions in NCERT Class 7 Maths Chapter 9 Perimeter and Area can vary from student to student. However, this chapter is generally considered to be of moderate difficulty. As per the revised syllabus and new textbooks issued for academic year 2024-25, there are only two exercises in chapter 9 of 7th mathematics. It involves concepts related to calculating the perimeter and area of various shapes.
7th Maths Chapter 9 Solutions in English Medium
7th Maths Chapter 9 Solutions in Hindi Medium
| Class: 7 | Mathematics |
| Chapter 9: | Perimeter and Area |
| Number of Exercises: | 2 (Two) |
| Study Material: | NCERT Exercises and Try These Solutions |
| Content Mode: | Online Text, Images and Videos Download |
| Academic Session: | 2024-25 |
| Medium: | English and Hindi Medium |
If you’re familiar with basic geometric shapes, their properties, and formulas for calculating perimeter and area, you might find this chapter easier to approach. Geometry involves visualizing shapes and their measurements. If you’re good at visualizing and drawing shapes accurately, you may find the solutions more manageable. The key to mastering this chapter is practice. As you solve more problems, you’ll become more comfortable with applying formulas and methods.
NCERT Solutions for Class 7 Maths Chapter 9
Pay attention to the units of measurement used in the solutions. Ensure that your answers have the correct units, whether it’s centimeters, meters, square centimeters, square meters, etc. Class VII Maths Exercise 9.1 and Exercise 9.2 in English Medium or Prashnavali 9.1 and Prashnavali 9.2 in Hindi Medium to study online or download in PDF file format. Understanding the formulas for calculating perimeter and area of different shapes is crucial. Make sure you know the formulas well and understand how they are derived. Latest NCERT (https://ncert.nic.in/) Books for 2024-25 and NCERT Solutions Apps for the new academic session are also available to free download without any registration. Some problems might be presented in the form of word problems. Focus on translating the information provided into mathematical expressions and equations.
7 Maths Chapter 9 Solutions
Drawing accurate diagrams is important for visualizing the shapes and measurements. Practice drawing diagrams that accurately represent the given information. Class 7 Maths Chapter 9 Perimeter and Area Exercise 9.1 and Exercise 9.2 with step by step proper answers and solutions are given below. Break down the problems step by step. Identify what’s given, what’s asked for, and the relevant formula or method to use. NCERT Solutions are updated for the new session 2024-25 based on new 7th NCERT Books. If you’re struggling with certain concepts or problems, look for online tutorials, videos, and interactive resources that explain the concepts in different ways.
Important Questions on Class 7 Maths Chapter 9
The length and breadth of a rectangular piece of land are 500 m and 300 m respectively. Find its area.
Given: Length of a rectangular piece of land = 500 m and
Breadth of a rectangular piece of land = 300 m
Area of a rectangular piece of land
= Length x Breadth = 500 x 300 = 1,50,000 m²
Find the area of a square park whose perimeter is 320 m.
Given: Perimeter of square park = 320 m
4 x side = 320
side = 320/4 = 80 m
Now, Area of square park = side x side = 80 x 80 = 6400 m²
Thus, the area of square park is 6400 m².
Find the breadth of a rectangular plot of land, if its area is 440 m² and the length is 22 m. Also find its perimeter.
Area of rectangular park = 440 m²
length x breadth = 440 m²
22 x breadth = 440
breadth = 440/22 = 20 m
Now, Perimeter of rectangular park
= 2 (length + breadth)
= 2 (22 + 20) = 2 x 42 = 84 m
Thus, the perimeter of rectangular park is 84 m.
The perimeter of a rectangular sheet is 100 cm. If the length is 35 cm, find its breadth. Also find the area.
Perimeter of the rectangular sheet
= 100 cm
2 (length + breadth) = 100 cm
2 (35 + breadth) = 100
35 + breadth = 100/2
35 + breadth = 50
breadth = 50 – 35
breadth = 15 cm
Now, Area of rectangular sheet
= length x breadth
= 35 x 15 = 525 cm²
Thus, breadth and area of rectangular sheet are 15 cm and 525 cm² respectively.
The perimeter of a rectangle is 130 cm. If the breadth of the rectangle is 30 cm, find its length. Also, find the area of the rectangle.
Perimeter of rectangle = 130 cm
2 (length + breadth) = 130 cm
2 (length + 30) = 130
length + 30 = 130/2
length + 30 = 65
length = 65 – 30 = 35 cm
Now area of rectangle
= length x breadth
= 35 x 30 = 1050 cm²
Thus, the area of rectangle is 1050 cm².
About NCERT Solutions for Class 7 Maths Chapter 9
In 7 Maths Chapter 9 Perimeter and Area, we have to go through calculation of perimeters of some basic figures like triangular region, square and some other simple figures. Area of parallelogram, area of triangle and area of triangle as a part of a quadrilateral, etc. We must consider the fact that all the congruent triangles are equal in area but the triangles equal in area need not be congruent. As we know that the distance around a circular region is known as its circumference, so the perimeter of a circular region is normally refer as circumference. Important formulae related to areas are given below:
1. Perimeter of a square = 4 × side
2. Perimeter of a rectangle = 2 × (length + breadth)
3. Area of a square = side × side
4. Area of a rectangle = length × breadth
5. Area of a parallelogram = base × height
6. Area of a triangle = ½ (area of the parallelogram generated from it) = ½ × base × height
7. Circumference of a circle = πd, where d is the diameter of a circle
8. Area of a circle = πr², where r is the radius of the circle.
9. The value of π can be taken 22/7 or 3.14 (approximately). Use any value if the value of π is not mention in the question.
About Class 7 Maths Chapter 9 Solutions
If you encounter difficulties, don’t hesitate to discuss problems with your classmates or teachers. Sometimes, a different perspective can help clarify things. Regularly review the formulas and practice solving problems. Repetition helps reinforce your understanding and memory.
Remember that every student’s learning curve is different. If you find certain problems or concepts challenging, that’s perfectly normal. Focus on understanding the underlying concepts, practice consistently, and don’t hesitate to seek help when needed. With effort and perseverance, you can overcome challenges and master the solutions in chapter 9 math.
Your Suggestions Our Strength
NCERT Solutions are being updated as per your suggestions or feedback. Approach the solutions with a positive attitude. Even if you find certain problems challenging initially, with practice and determination, you can improve your skills. Please notify us for even a single error. You can suggest also for the changes which makes website more user friendly for NCERT Books solutions and for offline Apps in the academic session 2024-25.